10.1:使用平方根属性求解二次方程
在本节结束时,您将能够:
- ax^2=k使用平方根属性求解形式的二次方程
- a(x−h)^2=k使用平方根属性求解形式的二次方程
在@@
- 简化:\sqrt{75}。
- 简化:\sqrt{\dfrac{64}{3}}
- 因子:4x^{2} − 12x + 9。
二次方程是形式的方程式ax^{2} + bx + c = 0,其中a \neq 0。 它们与线性方程的不同之处在于,它们包含一个将变量提高到二次幂的项。 我们使用与线性方程不同的方法来求解二次方程 s,因为仅仅相加、减去、乘和除项并不能隔离变量。
我们已经看到,一些二次方程可以通过分解来求解。 在本章中,我们将使用其他三种方法来求解二次方程。
ax^2=k使用平方根属性求解形式的二次方程
我们已经通过分解求解了一些二次方程。 让我们回顾一下我们是如何使用分解来求解二次方程的x^{2} = 9。
\begin{array}{ll} {}&{x^2=9}\\ {\text{Put the equation in standard form.}}&{x^2−9=0}\\ {\text{Factor the left side.}}&{(x - 3)(x + 3) = 0}\\ {\text{Use the Zero Product Property.}}&{(x - 3) = 0, (x + 3) = 0}\\ {\text{Solve each equation.}}&{x = 3, x = -3}\\ {\text{Combine the two solutions into} \pm \text{form}}&{x=\pm 3}\\ \nonumber \end{array}
(解读x为 “等于正或负”3。)
我们可以很容易地使用因子分解来找到相似方程的解,比如x^{2}=16和x^{2} = 25,因为16和25是完美的正方形。 但是当我们有这样的方程式时会发生什么x^{2}=7? 由于7不是完美的正方形,因此我们无法通过分解来求解方程。
这些方程都是形式上的x^{2}=k。
我们用这种方式定义了数字的平方根:
如果n^{2} = m,n则为的平方根m。
这就产生了平方根属性。
如果x^{2}=k、和k \geq 0、然后x = \sqrt{k}或x = -\sqrt{k}。
请注意,平方根属性为形式的方程给出了两个解x^2=k:k 的主平方根及其对立面。 我们也可以将解决方案写成x=\pm \sqrt{k}
现在,我们将x^{2} = 9再次求解方程,这次是使用平方根属性。
\begin{array}{ll} {}&{x^{2} = 9}\\ {\text{Use the Square Root Property.}}&{x = \pm\sqrt{9}}\\ {\text{Simplify the radical.}}&{x = \pm 3}\\ {\text{Rewrite to show the two solutions.}}&{x = 3, x = −3}\\ \nonumber \end{array}
当常量不是完美正方形时会发生什么? 让我们使用平方根属性来求解方程x^2=7。
\begin{array} {ll} {\text{Use the Square Root Property. }}&{x = \pm\sqrt{7}}\\ {\text{Rewrite to show two solutions.}}&{x = \sqrt{7}, x = −\sqrt{7}}\\ {\text{We cannot simplify} \sqrt{7} \text{ so we leave the answer as a radical.}}&{}\\ \nonumber \end{array}
解决:x^{2} = 169
- 回答
-
\begin{array}{ll} {}&{x^2=169}\\ {\text{Use the Square Root Property.}}&{x=\pm\sqrt{169}}\\ {\text{Simplify the radical.}}&{x = \pm13}\\{\text{Rewrite to show two solutions.}}&{x = 13, x = −13}\\ \nonumber \end{array}
解决:x^2=81
- 回答
-
x=9,x=−9
解决:y^{2} = 121
- 回答
-
y = 11,y = −11
如何ax^{2} = k使用平方根属性求解这种形式的二次方程
解决:x^{2} − 48 = 0
- 回答



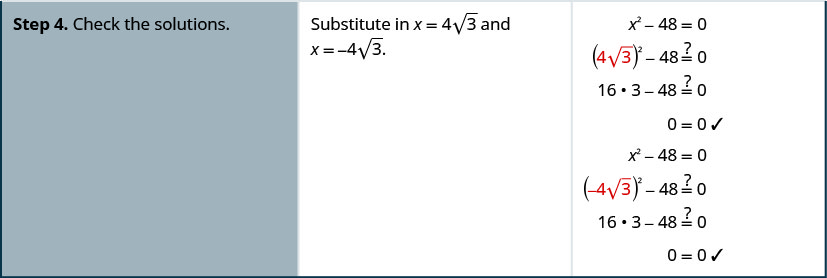
解决:x^{2} − 50 = 0
- 回答
-
x = 5\sqrt{2}, x = −5\sqrt{2}
解决:y^{2} − 27 = 0
- 回答
-
y = 3\sqrt{3}, x = −3\sqrt{3}
- 分离二次项并将其系数设为一。
- 使用平方根属性。
- 简化激进。
- 检查解决方案。
要使用平方根属性,变量项的系数必须等于 1。 在下一个示例中,在使用平方根属性之前,我们必须将方程的两边除以 5。
解决:5m^2=80
- 回答
-
二次项是孤立的。 5m^2=80 除以 5 得出 cofficient 1。 \frac{5m^2}{5}=\frac{80}{5} 简化。 m^2=16 使用平方根属性。 m=\pm\sqrt{16} 简化激进。 m=\pm 4 重写以显示两个解决方案。 m=4,m=−4 检查解决方案。
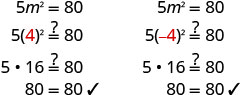
解决:2x^2=98。
- 回答
-
x=7,x=−7
解决:3z^2=108。
- 回答
-
z=6,z=−6
平方根属性以表示 “x^2=kIf ank\ge 0 d” 开头。 如果会发生什么k<0? 在下一个示例中,情况将如此。
解决:q^2+24=0。
- 回答
-
\begin{array}{ll} {}&{q^2=24}\\ {\text{Isolate the quadratic term.}}&{q^2=−24}\\ {\text{Use the Square Root Property.}}&{q=\pm\sqrt{-24}}\\ {\text{The} \sqrt{-24} \text{is not a real number}}& {\text{There is no real solution}}\\ \nonumber \end{array}
解决:c^2+12=0。
- 回答
-
没有真正的解决方案
解决:d^2+81=0。
- 回答
-
没有真正的解决方案
解决:\frac{2}{3}u^2+5=17。
- 回答
-
\frac{2}{3}u^2+5=17 分离二次项。 \frac{2}{3}u^2=12
乘\frac{3}{2}以得出系数 1。 \frac{3}{2}·\frac{2}{3}u^2=\frac{3}{2}·12 简化。 u^2=18 使用平方根属性。 u=\pm\sqrt{18} 简化激进。 u=\pm\sqrt{9}\sqrt{2} 简化。 u=\pm3\sqrt{2} 重写以显示两个解决方案。 u=3\sqrt{2},u=−3\sqrt{2} 查看。

解决:\frac{1}{2}x^2+4=24
- 回答
-
x=2\sqrt{10},x=−2\sqrt{10}
解决:\frac{3}{4}y^2−3=18。
- 回答
-
y=2\sqrt{7},y=−2\sqrt{7}
某些方程的解在自由基内部可能有分数。 当这种情况发生时,我们必须使分母合理化。
解决:2c^2−4=45。
- 回答
-
2c^2−4=45 分离二次项。 2c^2=49 除以 2 得出系数 1。 \frac{2c^2}{2}=\frac{49}{2} 简化。 c^2=\frac{49}{2} 使用平方根属性。 c=\pm\frac{\sqrt{49}}{\sqrt{2}} 简化激进。 c=\pm\frac{\sqrt{49}}{\sqrt{2}} 合理化分母。 c=\pm\frac{\sqrt{49}\sqrt{2}}{\sqrt{2}\sqrt{2}} 简化。 c=\pm\frac{7\sqrt{2}}{2} 重写以显示两个解决方案。 c=\frac{7\sqrt{2}}{2},c=−\frac{7\sqrt{2}}{2} 查看。 我们把支票留给你。
解决:5r^2−2=34。
- 回答
-
r=\frac{6\sqrt{5}}{5},r=−\frac{6\sqrt{5}}{5}
解决:3t^2+6=70。
- 回答
-
t=\frac{8\sqrt{3}}{3},t=−\frac{8\sqrt{3}}{3}
a(x-h)^2=k使用平方根属性求解形式的二次方程
我们也可以使用平方根属性来求解方程。(x−3)^2=16 我们将整个二项式 (x−3) 视为二次项。
解决:(x−3)^2=16。
- 回答
-
(x−3)^2=16 使用平方根属性。 x−3=\pm\sqrt{16} 简化。 x−3=\pm 4 写成两个方程式。 x−3=4,x−3=−4 解决。 x=7,x=−1 查看。
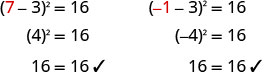
解决:(q+5)^2=1。
- 回答
-
q=−6,q=−4
解决:(r−3)^2=25。
- 回答
-
r=8,r=−2
解决:(y−7)^2=12。
- 回答
-
(y−7)^2=12。 使用平方根属性。 y−7=\pm\sqrt{12} 简化激进。 y−7=\pm2\sqrt{3} 求解 y。 y=7\pm2\sqrt{3} 重写以显示两个解决方案。 y=7+2\sqrt{3},y=7−2\sqrt{3} 查看。

解决:(a−3)^2=18。
- 回答
-
a=3+3\sqrt{2},a=3−3\sqrt{2}
解决:(b+2)^2=40。
- 回答
-
b=−2+2\sqrt{10},b=−2−2\sqrt{10}
解决:(x−\frac{1}{2})^2=\frac{5}{4}。
- 回答
-
(x−\frac{1}{2})^2=\frac{5}{4} 使用平方根属性。 (x−\frac{1}{2})=\pm\sqrt\frac{5}{4} 将基数重写为平方根的分数。 (x−\frac{1}{2})=\pm\frac{\sqrt{5}}{\sqrt{4}} 简化激进。 (x−\frac{1}{2})=\pm\frac{\sqrt{5}}{2} 求解 x。 x=\frac{1}{2}+\pm\frac{\sqrt{5}}{2} 重写以显示两个解决方案。 x=\frac{1}{2}+\frac{\sqrt{5}}{2},x=\frac{1}{2}−\frac{\sqrt{5}}{2} 查看。 我们把支票留给你
解决:(x−\frac{1}{3})^2=\frac{5}{9}。
- 回答
-
x=\frac{1}{3}+\frac{\sqrt{5}}{3},x=\frac{1}{3}−\frac{\sqrt{5}}{3}
解决:(y−\frac{3}{4})^2=\frac{7}{16}。
- 回答
-
y=\frac{3}{4}+\frac{\sqrt{7}}{4},y=\frac{3}{4}−\frac{\sqrt{7}}{4},
我们将通过隔离二项式来开始下一个示例的解。
解决:(x−2)^2+3=30。
- 回答
-
(x−2)^2+3=30 分离二项式项。 (x−2)^2=27 使用平方根属性。 x−2=\pm\sqrt{27} 简化激进。 x−2=\pm3\sqrt{3} 求解 x。 x=2+\pm3\sqrt{3} x−2=\pm3\sqrt{3} x=2+3\sqrt{3},x=2−3\sqrt{3} 查看。 我们把支票留给你
解决:(a−5)^2+4=24。
- 回答
-
a=5+2\sqrt{5},a=5−2\sqrt{5}
解决:(b−3)^2−8=24。
- 回答
-
b=3+4\sqrt{2},b=3−4\sqrt{2}
解决:(3v−7)^2=−12。
- 回答
-
(3v−7)^2=−12 使用平方根属性。 3v−7=\pm\sqrt{−12} \sqrt{−12}不是实数。 没有真正的解决方案。
解决:(3r+4)^2=−8。
- 回答
-
没有真正的解决方案
在接下来的两个例子中,方程的左边似乎不是这种形式a(x−h)^2。 但是它们是完美的正方三项式,所以我们会考虑把它们变成我们需要的形式。
解决:p^2−10p+25=18。
- 回答
-
方程的左侧是一个完美的方形三项式。 我们将首先将其考虑在内。
p^2−10p+25=18 将完美的正方三项式分解出来。 (p−5)^2=18 使用平方根属性。 p−5=\pm\sqrt{18} 简化激进。 p−5=\pm3\sqrt{2} 求解 p。 p=5\pm3\sqrt{2} 重写以显示两个解决方案。 p=5+3\sqrt{2},p=5−3\sqrt{2} 查看。 我们把支票留给你。
解决:x^2−6x+9=12。
- 回答
-
x=3+2\sqrt{3},x=3−2\sqrt{3}
解决:y^2+12y+36=32。
- 回答
-
y=−6+4\sqrt{2},y=−6−4\sqrt{2}
解决:4n^2+4n+1=16。
- 回答
-
同样,我们注意到方程的左侧是一个完美的方形三项式。 我们将首先将其考虑在内。
4n^2+4n+1=16 将完美的正方三项式分解出来。 (2n+1)^2=16 使用平方根属性。 (2n+1)=\pm\sqrt{16} 简化激进。 (2n+1)=\pm4 求解 n。 2n=−1\pm4 将每边除以 2。 \frac{2n}{2}=\frac{−1\pm4}{2}
n=\frac{−1\pm4}{2}
重写以显示两个解决方案。 n=\frac{−1+4}{2},n=\frac{−1−4}{2} 简化每个方程。 n=\frac{3}{2},n=−\frac{5}{2} 查看。

解决:9m^2−12m+4=25。
- 回答
-
m=\frac{7}{3},m=−1
解决:16n^2+40n+25=4。
- 回答
-
n=−\frac{3}{4}, n=−\frac{7}{4}
访问以下在线资源,获取有关求解二次方程的更多指导和练习:
- 求解二次方程:取平方根求解
- 使用平方根求解二次方程
- 求解二次方程:平方根法
关键概念
- 平方根属性 I
fx^2=k、andk\ge 0、thenx=\sqrt{k} orx=−\sqrt{k}。
词汇表
- 二次方程
- 二次方程是形式ax^2+bx+c=0为 where 的方程a \ne 0。
- 平方根属性
- 平方根属性指出 if、anx^2=k dk\ge 0、thenx=\sqrt{k} orx=−\sqrt{k}。


