6.5: 除以单项式
- Page ID
- 204955
在本节结束时,您将能够:
- 使用指数的商属性简化表达式
- 使用零指数简化表达式
- 使用商为幂属性简化表达式
- 通过应用多个属性来简化表达式
- 划分单项式
使用指数的商属性简化表达式
在本章的前面,我们开发了乘法指数的属性。 我们在下面总结了这些属性。
如果 a 和 b 是实数,而 m 和 n 是整数,那么
\[\begin{array}{ll}{\textbf { Product Property }} & {a^{m} \cdot a^{n}=a^{m+n}} \\ {\textbf { Power Property }} & {\left(a^{m}\right)^{n}=a^{m n}} \\ {\textbf { Product to a Power }} & {(a b)^{m}=a^{m} b^{m}}\end{array}\]
现在我们来看看除法的指数属性。 在我们开始之前,快速刷新内存可能会有所帮助。 你已经学会了通过使用等效分数属性从分子和分母中除去常见因子来简化分数。 这个属性还将帮助你处理代数分数,代数分数也是商。
如果 a、b 和 c 是整数,其中\(b\neq 0,c\neq 0\)。
\[\text{then} \quad \dfrac{a}{b}=\dfrac{a \cdot c}{b \cdot c} \quad \text{and} \quad \dfrac{a \cdot c}{b \cdot c}=\dfrac{a}{b}\]
和以前一样,我们将尝试通过查看一些示例来发现房产。
\[\begin{array}{lclc}{\text { Consider }} & \dfrac{x^{5}}{x^{2}} & \text{and} & \dfrac{x^{2}}{x^{3}}\\ {\text { What do they mean? }}&\dfrac{x \cdot x \cdot x \cdot x \cdot x}{x \cdot x} && \dfrac{x \cdot x}{x \cdot x \cdot x}\\ {\text { Use the Equivalent Fractions Property. }} & {\dfrac{x \not\cdot x \not\cdot x \cdot x \cdot x}{x \not\cdot\not x}} && \dfrac{\not x \cdot\not x \cdot 1}{x \not \cdot\not x \cdot x}\\ {\text { Simplify. }} & {x^{3}} & & \dfrac{1}{x}\end{array}\]
注意,在每种情况下,基数都是一样的,我们减去了指数。
当分子中出现较大的指数时,分子中就剩下了因子。
当分母中出现较大的指数时,我们在分母中就剩下了因子——注意 1 的分子。
我们写道:
\[\begin{array}{cc}{\dfrac{x^{5}}{x^{2}}} & {\dfrac{x^{2}}{x^{3}}} \\ {x^{5-2}} & {\dfrac{1}{x^{3-2}}} \\ {x^{3}} & {\dfrac{1}{x}}\end{array}\]
这就产生了指数的商属性。
如果 a 是实数\(a\neq 0\),而 m 和 n 是整数,那么
\[\dfrac{a^{m}}{a^{n}}=a^{m-n}, m>n \text { and } \dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{n-m}}, n>m\]
举几个带数字的例子可能有助于验证这个属性。
\[\begin{array} {llllll} \dfrac{3^{4}}{3^{2}} &=&3^{4-2}& \dfrac{5^{2}}{5^{3}} &=&\dfrac{1}{5^{3-2}} \\ \dfrac{81}{9} &=&3^{2} & \dfrac{25}{125} &=&\dfrac{1}{5^{1}} \\ 9 &=&9\checkmark& \dfrac{1}{5} &=&\dfrac{1}{5} \checkmark \end{array}\]
简化:
- \(\dfrac{x^{9}}{x^{7}}\)
- \(\dfrac{3^{10}}{3^{2}}\)
- 回答
-
为了简化带商的表达式,我们需要首先比较分子和分母中的指数。
1。
由于 9 > 7,分子中有 x 的因子更多。 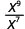
使用商数属性,\(\dfrac{a^{m}}{a^{n}}=a^{m-n}\) 
简化。 \(x^2\) 2。
请注意,当分子中存在较大的指数时,分子中就剩下了因子。由于 10 > 2,分子中有 x 的因子更多。 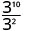
使用商数属性,\(\dfrac{a^{m}}{a^{n}}=a^{m-n}\) 
简化。 \(3^8\)
简化:
- \(\dfrac{x^{15}}{x^{10}}\)
- \(\dfrac{6^{14}}{6^{5}}\)
- 回答
-
- \(x^{5}\)
- \(6^9\)
简化:
- \(\dfrac{y^{43}}{y^{37}}\)
- \(\dfrac{10^{15}}{10^{7}}\)
- 回答
-
- \(y^{6}\)
- \(10^8\)
简化:
- \(\dfrac{b^{8}}{b^{12}}\)
- \(\dfrac{7^{3}}{7^{5}}\)
- 回答
-
为了简化带商的表达式,我们需要首先比较分子和分母中的指数。
1。
由于 12 > 8,分母中有更多的 b 因子。 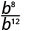
使用商数属性,\(\dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{n-m}}\) 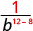
简化。 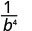
2。
请注意,当分母中存在较大的指数时,分母中就剩下了因子。由于 5 > 3,分母中有更多因子 3。 
使用商数属性,\(\dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{n-m}}\) 
简化。 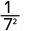
简化。 
简化:
- \(\dfrac{x^{18}}{x^{22}}\)
- \(\dfrac{12^{15}}{12^{30}}\)
- 回答
-
- \(\dfrac{1}{x^{4}}\)
- \(\dfrac{1}{12^{15}}\)
简化:
- \(\dfrac{m^{7}}{m^{15}}\)
- \(\dfrac{9^{8}}{9^{19}}\)
- 回答
-
- \(\dfrac{1}{m^{8}}\)
- \(\dfrac{1}{9^{11}}\)
请注意前两个示例的区别:
- 如果我们从分子中的更多因子开始,则分子中的因子最终会出现。
- 如果我们从分母中的更多因子开始,我们最终会得到分母中的因子。
使用指数的商属性简化表达式的第一步是确定指数在分子还是分母中更大。
简化:
- \(\dfrac{a^{5}}{a^{9}}\)
- \(\dfrac{x^{11}}{x^{7}}\)
- 回答
-
1。 分子或分母中a的指数是否较大? 由于 9 > 5,分母中有更多的 a,所以我们最终会得到分母中的因子。
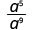
使用商数属性,\(\dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{n-m}}\) 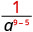
简化。 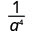
2。 注意分子中有 xx 的更多因子,因为 11 > 7。 因此,我们最终将在分子中得到因子。
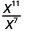
使用商数属性,\(\dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{n-m}}\) 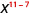
简化。 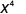
简化:
- \(\dfrac{b^{19}}{b^{11}}\)
- \(\dfrac{z^{5}}{z^{11}}\)
- 回答
-
- \(b^{8}\)
- \(\dfrac{1}{z^{6}}\)
简化:
- \(\dfrac{p^{9}}{p^{17}}\)
- \(\dfrac{w^{13}}{w^{9}}\)
- 回答
-
- \(\dfrac{1}{p^{8}}\)
- \(w^{4}\)
使用零指数简化表达式
Quotient Property 的一个特例是分子和分母的指数相等,例如像这样的表达式\(\dfrac{a^{m}}{a^{m}}\)。 从你之前的分数研究中,你知道:
\[\dfrac{2}{2}=1 \quad \dfrac{17}{17}=1 \quad \dfrac{-43}{-43}=1\]
用文字表示,数字除以自身为 1。 因此\(\dfrac{x}{x}=1\),对于任何\(x(x\neq 0)\),因为任何数字除以自身都是 1。
指数的商属性向我们展示了如何\(n<m\)通过减去指数来简化\(\dfrac{a^{m}}{a^{n}}\)时间\(m>n\)和时间。 如果呢\(m=n\)?
考虑\(\dfrac{8}{8}\)一下,我们知道的是 1。
\(\begin{array} {lrll} & \dfrac{8}{8} &=&1 \\ \text { Write } 8 \text { as } 2^{3} . & \dfrac{2^{3}}{2^{3}} &=&1 \\ \text { Subtract exponents. } & 2^{3-3} &=&1 \\ \text { Simplify. } & 2^{0} &=&1 \end{array}\)
现在,我们将通过两种\(\dfrac{a^{m}}{a^{m}}\)方式进行简化,引导我们了解零指数的定义。 一般来说,用于\(a\neq 0\):
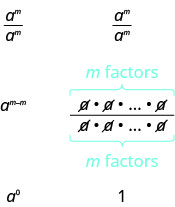
我们看到\(\dfrac{a^{m}}{a^{m}}\)简化为 1\(a^{0}\) 和 1。 所以\(a^{0} = 1\)。
如果 a 是非零数字,那么\(a^{0} = 1\)。
任何提高到零幂的非零数均为 1。
在本文中,我们假设我们提高到零次方的任何变量都不是零。
简化:
- \(9^{0}\)
- \(n^{0}\)
- 回答
-
定义是任何提高到零幂的非零数都是 1。
- \(\begin{array}{ll} & 9^0\\ \text{Use the definition of the zero exponent.} & 1 \end{array}\)
- \(\begin{array}{ll} & n^0\\ \text{Use the definition of the zero exponent.} & 1 \end{array}\)
简化:
- \(15^{0}\)
- \(m^{0}\)
- 回答
-
- 1
- 1
简化:
- \(k^{0}\)
- \(29^{0}\)
- 回答
-
- 1
- 1
现在我们已经定义了零指数,我们可以扩展指数的所有属性以包括整数指数。
将表达式提高到零幂怎么样? 让我们来看看\((2x)^0\)。 我们可以使用乘积乘法则来重写这个表达式。
\[\begin{array}{ll} & (2x)^0\\ {\text { Use the product to a power rule. }} & {2^{0} x^{0}} \\ {\text { Use the zero exponent property. }} & {1 \cdot 1} \\ {\text { Simplify. }} & 1\end{array}\]
这告诉我们,任何提高到零幂的非零表达式都是一个。
简化:
- \((5b)^0\)
- \((−4a^{2}b)^0\)。
- 回答
-
- \(\begin{array}{ll} & (5b)^0\\ {\text {Use the definition of the zero exponent.}} & 1\end{array}\)
- \(\begin{array}{ll} & (−4a^{2}b)^0\\ {\text {Use the definition of the zero exponent.}} & 1\end{array}\)
简化:
- \((11z)^0\)
- \((−11pq^{3})^0\)。
- 回答
-
- 1
- 1
简化:
- \((-6d)^0\)
- \((−8m^{2}n^{3})^0\)。
- 回答
-
- 1
- 1
使用商乘方属性简化表达式
现在我们来看一个例子,它将引导我们找到权属性的商。
\[\begin{array}{lc} & {\left(\dfrac{x}{y}\right)^{3}} \\ \text{This means:} & {\dfrac{x}{y} \cdot \dfrac{x}{y} \cdot \dfrac{x}{y}} \\ \text{Multiply the fractions.} &{\dfrac{x \cdot x \cdot x}{y \cdot y \cdot y}} \\ \text{Write with exponents.} & {\dfrac{x^{3}}{y^{3}}}\end{array}\]
请注意,指数既适用于分子,也适用于分母。
\[\begin{array}{lc}{\text { We see that }\left(\dfrac{x}{y}\right)^{3} \text { is } \dfrac{x^{3}}{y^{3}}} \\ {\text { We write: }} & \left(\dfrac{x}{y}\right)^{3} \\ & {\dfrac{x^{3}}{y^{3}}} \end{array}\]
这会导致指数的商变为幂属性。
如果 a 和 b 是实数\(b\neq 0\),而 m 是计数数,那么
\[\left(\dfrac{a}{b}\right)^{m}=\dfrac{a^{m}}{b^{m}}\]
要将分数提高到乘方,请将分子和分母提高到该次方。
一个带有数字的例子可以帮助你理解这个属性:
\[\begin{aligned}\left(\dfrac{2}{3}\right)^{3} &=\dfrac{2^{3}}{3^{3}} \\ \dfrac{2}{3} \cdot \dfrac{2}{3} \cdot \dfrac{2}{3} &=\dfrac{8}{27} \\ \dfrac{8}{27} &=\dfrac{8}{27}\checkmark \end{aligned}\]
简化:
- \(\left(\dfrac{3}{7}\right)^{2}\)
- \(\left(\dfrac{b}{3}\right)^{4}\)
- \(\left(\dfrac{k}{j}\right)^{3}\)
- 回答
-
1。
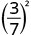
使用商数属性,\(\left(\dfrac{a}{b}\right)^{m}=\dfrac{a^{m}}{b^{m}}\) 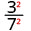
简化。 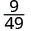
2。
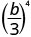
使用商数属性,\(\left(\dfrac{a}{b}\right)^{m}=\dfrac{a^{m}}{b^{m}}\) 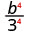
简化。 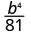
3。
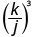
将分子和分母提高到三次方。 
简化:
- \(\left(\dfrac{5}{8}\right)^{2}\)
- \(\left(\dfrac{p}{10}\right)^{4}\)
- \(\left(\dfrac{m}{n}\right)^{7}\)
- 回答
-
- \(\dfrac{25}{64}\)
- \(\dfrac{p^{4}}{10,000}\)
- \(\dfrac{m^{7}}{n^{7}}\)
简化:
- \(\left(\dfrac{1}{3}\right)^{3}\)
- \(\left(\dfrac{-2}{q}\right)^{3}\)
- \(\left(\dfrac{w}{x}\right)^{4}\)
- 回答
-
- \(\dfrac{1}{27}\)
- \(\dfrac{-8}{q^{3}}\)
- \(\dfrac{w^{4}}{x^{4}}\)
通过应用多个属性来简化表达式
现在,我们将总结指数的所有属性,以便在我们使用多个属性简化表达式时将它们一起引用。 请注意,它们现在是为整数指数定义的。
如果 a 和 b 是实数,而 m 和 n 是整数,那么
\[\begin{array}{lrll} \textbf{Product Property} & a^{m} \cdot a^{n} &=&a^{m+n} \\\textbf{Power Property} & \left(a^{m}\right)^{n} &=&a^{m \cdot n} \\\textbf{Product to a Power} & (a b)^{m} &=&a^{m} b^{m} \\ \textbf{Quotient Property} & \dfrac{a^{m}}{a^{n}} &=&a^{m-n}, a \neq 0, m>n \\ & \dfrac{a^{n}}{a^{n}} &=&1, a \neq 0, n>m \\\textbf{Zero Exponent Definition} &a^0&=&1,a\neq 0 \\\textbf{Quotient to a Power Property} & \left(\dfrac{a}{b}\right)^{m} &=&\dfrac{a^{m}}{b^{m}}, b \neq 0 \end{array}\]
简化:\(\dfrac{\left(y^{4}\right)^{2}}{y^{6}}\)
- 回答
-
\(\begin{array} {ll} & \dfrac{\left(y^{4}\right)^{2}}{y^{6}} \\ \text{Multiply the exponents in the numerator.} & \dfrac{y^{8}}{y^{6}}\\ \text{Subtract the exponents.} &y^{2} \end{array}\)
简化:\(\dfrac{\left(m^{5}\right)^{4}}{m^{7}}\)
- 回答
-
\(m^{13}\)
简化:\(\dfrac{\left(k^{2}\right)^{6}}{k^{7}}\)
- 回答
-
\(k^{5}\)
简化:\(\dfrac{b^{12}}{\left(b^{2}\right)^{6}}\)
- 回答
-
\[\begin{array} {ll} &\dfrac{b^{12}}{\left(b^{2}\right)^{6}} \\ \text{Multiply the exponents in the numerator.} & \dfrac{b^{12}}{b^{12}}\\ \text{Subtract the exponents.} &b^{0} \\ \text{Simplify} & 1\end{array}\]
请注意,我们在第一步中简化了分母之后,分子和分母是相等的。 因此,最终值等于 1。
简化\(\dfrac{n^{12}}{\left(n^{3}\right)^{4}}\)。
- 回答
-
1
简化\(\dfrac{x^{15}}{\left(x^{3}\right)^{5}}\)。
- 回答
-
1
简化:\(\left(\dfrac{y^{9}}{y^{4}}\right)^{2}\)
- 回答
-
\[\begin{array} {ll} &\left(\dfrac{y^{9}}{y^{4}}\right)^{2}\\ \text{Remember parentheses come before exponents.} &\\ \text{Notice the bases are the same, so we can simplify} &\left(y^{5}\right)^{2} \\ \text{inside the parentheses. Subtract the exponents.} & \\\text{Multiply the exponents.} &y^{10} \end{array}\]
简化:\(\left(\dfrac{r^{5}}{r^{3}}\right)^{4}\)
- 回答
-
\(r^{8}\)
简化:\(\left(\dfrac{v^{6}}{v^{4}}\right)^{3}\)
- 回答
-
\(v^{6}\)
简化:\(\left(\dfrac{j^{2}}{k^{3}}\right)^{4}\)
- 回答
-
这里我们不能先简化括号内的内容,因为基数不一样。
\(\begin{array} {ll} &\left(\dfrac{j^{2}}{k^{3}}\right)^{4}\\ \text{Raise the numerator and denominator to the third power} & \\ \text{using the Quotient to a Power Property,}\left(\dfrac{a}{b}\right)^{m}=\dfrac{a^{m}}{b^{m}} &\dfrac{\left(j^{2}\right)^{4}}{\left(k^{3}\right)^{4}}\\ \text{Use the Power Property and simplify.} & \dfrac{j^{8}}{k^{12}} \end{array}\)
简化:\(\left(\dfrac{a^{3}}{b^{2}}\right)^{4}\)
- 回答
-
\(\dfrac{a^{12}}{b^{8}}\)
简化:\(\left(\dfrac{q^{7}}{r^{5}}\right)^{3}\)
- 回答
-
\(\dfrac{q^{21}}{r^{15}}\)
简化:\(\left(\dfrac{2 m^{2}}{5 n}\right)^{4}\)
- 回答
-
\(\begin{array} {ll} &\left(\dfrac{2 m^{2}}{5 n}\right)^{4}\\ \text{Raise the numerator and denominator to the fourth} &\dfrac{\left(2 m^{2}\right)^{4}}{(5 n)^{4}} \\ \text{power, using the Quotient to a Power Property,}\left(\dfrac{a}{b}\right)^{m}=\dfrac{a^{m}}{b^{m}} &\dfrac{2^{4}\left(m^{2}\right)^{4}}{5^{4} n^{4}}\\ \text{Use the Power Property and simplify.} & \dfrac{16 m^{8}}{625 n^{4}} \end{array}\)
简化:\(\left(\dfrac{7 x^{3}}{9 y}\right)^{2}\)
- 回答
-
\(\dfrac{49 x^{6}}{81 y^{2}}\)
简化:\(\left(\dfrac{3 x^{4}}{7 y}\right)^{2}\)
- 回答
-
\(\dfrac{9 x^{8}}{49 v^{2}}\)
简化:\(\dfrac{\left(x^{3}\right)^{4}\left(x^{2}\right)^{5}}{\left(x^{6}\right)^{5}}\)
- 回答
-
\(\begin{array}{ll}&\dfrac{\left(x^{3}\right)^{4}\left(x^{2}\right)^{5}}{\left(x^{6}\right)^{5}}\\ \text{Use the Power Property,}\left(a^{m}\right)^{n}=a^{m \cdot n} &\dfrac{\left(x^{12}\right)\left(x^{10}\right)}{\left(x^{30}\right)}\\ \text{Add the exponents in the numerator.} &\dfrac{x^{22}}{x^{30}}\\ \text{Use the Quotient Property,} \dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{n-m}}&\dfrac{1}{x^{8}}\end{array}\)
简化:\(\dfrac{\left(a^{2}\right)^{3}\left(a^{2}\right)^{4}}{\left(a^{4}\right)^{5}}\)
- 回答
-
\(\dfrac{1}{a^{6}}\)
简化:\(\dfrac{\left(p^{3}\right)^{4}\left(p^{5}\right)^{3}}{\left(p^{7}\right)^{6}}\)
- 回答
-
\(\dfrac{1}{p^{15}}\)
简化:\(\dfrac{\left(10 p^{3}\right)^{2}}{(5 p)^{3}\left(2 p^{5}\right)^{4}}\)
- 回答
-
\(\begin{array} {ll} &\dfrac{\left(10 p^{3}\right)^{2}}{(5 p)^{3}\left(2 p^{5}\right)^{4}}\\ \text { Use the Product to a Power Property, }(a b)^{m}=a^{m} b^{m}&\dfrac{(10)^{2}\left(p^{3}\right)^{2}}{(5)^{3}(p)^{3}(2)^{4}\left(p^{5}\right)^{4}}\\ \text { Use the Power Property, }\left(a^{m}\right)^{n}=a^{m \cdot n}&\dfrac{100 p^{6}}{125 p^{3} \cdot 16 p^{20}}\\ \text { Add the exponents in the denominator. }&\dfrac{100 p^{6}}{125 \cdot 16 p^{23}} \\ \text { Use the Quotient Property, } \dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{n-m}} & \dfrac{100}{125 \cdot 16 p^{17}} \\ \text { Simplify. } & \dfrac{1}{20 p^{17}} \end{array}\)
简化:\(\dfrac{\left(3 r^{3}\right)^{2}\left(r^{3}\right)^{7}}{\left(r^{3}\right)^{3}}\)
- 回答
-
9\(r^{18}\)
简化:\(\dfrac{\left(2 x^{4}\right)^{5}}{\left(4 x^{3}\right)^{2}\left(x^{3}\right)^{5}}\)
- 回答
-
\(\dfrac{2}{x}\)
划分单项式
现在,您已经了解了指数的所有属性,并使用它们来简化表达式。 接下来,您将看到如何使用这些属性来划分单项式。 稍后,您将使用它们来除多项式。
找到商:\(56 x^{7} \div 8 x^{3}\)
- 回答
-
\[\begin{array} {ll} &56 x^{7} \div 8 x^{3}\\ \text { Rewrite as a fraction. }&\dfrac{56 x^{7}}{8 x^{3}}\\ \text { Use fraction multiplication. }&\dfrac{56}{8} \cdot \dfrac{x^{7}}{x^{3}}\\ \text { Simplify and use the Quotient Property. }&7 x^{4}\end{array}\]
找到商:\(42y^{9} \div 6 y^{3}\)
- 回答
-
\(7y^{6}\)
找到商:\(48z^{8} \div 8 z^{2}\)
- 回答
-
\(6z^{6}\)
找到商:\(\dfrac{45 a^{2} b^{3}}{-5 a b^{5}}\)
- 回答
-
当我们用多个变量除以单项式时,我们为每个变量写一个分数。
\(\begin{array} {ll} &\dfrac{45 a^{2} b^{3}}{-5 a b^{5}}\\ \text { Use fraction multiplication. }&\dfrac{45}{-5} \cdot \dfrac{a^{2}}{a} \cdot \dfrac{b^{3}}{b^{5}}\\\text { Simplify and use the Quotient Property. }&-9 \cdot a \cdot \dfrac{1}{b^{2}}\\\text { Multiply. }&-\dfrac{9 a}{b^{2}}\end{array}\)
找到商:\(\dfrac{-72 a^{7} b^{3}}{8 a^{12} b^{4}}\)
- 回答
-
\(-\dfrac{9}{a^{5} b}\)
找到商:\(\dfrac{-63 c^{8} d^{3}}{7 c^{12} d^{2}}\)
- 回答
-
\(\dfrac{-9 d}{c^{4}}\)
找到商:\(\dfrac{24 a^{5} b^{3}}{48 a b^{4}}\)
- 回答
-
\(\begin{array} {ll} &\dfrac{24 a^{5} b^{3}}{48 a b^{4}}\\ \text { Use fraction multiplication. }&\dfrac{24}{48} \cdot \dfrac{a^{5}}{a} \cdot \dfrac{b^{3}}{b^{4}}\\\text { Simplify and use the Quotient Property. }&\dfrac{1}{2} \cdot a^{4} \cdot \dfrac{1}{b}\\\text { Multiply. }&\dfrac{a^{4}}{2 b}\end{array}\)
找到商:\(\dfrac{16 a^{7} b^{6}}{24 a b^{8}}\)
- 回答
-
\(\dfrac{2 a^{6}}{3 b^{2}}\)
找到商:\(\dfrac{27 p^{4} q^{7}}{-45 p^{12} q}\)
- 回答
-
\(-\dfrac{3 q^{6}}{5 p^{8}}\)
一旦你熟悉了这个过程并逐步练习了好几次,你也许可以一步简化一小部分。
找到商:\(\dfrac{14 x^{7} y^{12}}{21 x^{11} y^{6}}\)
- 回答
-
要非常小心,\(\dfrac{14}{21}\)通过除去一个公共因子来简化变量,并通过减去它们的指数来简化变量。
\(\begin{array} {ll} &\dfrac{14 x^{7} y^{12}}{21 x^{11} y^{6}}\\ \text { Simplify and use the Quotient Property. } & \dfrac{2 y^{6}}{3 x^{4}}\end{array}\)
找到商:\(\dfrac{28 x^{5} y^{14}}{49 x^{9} y^{12}}\)
- 回答
-
\(\dfrac{4 y^{2}}{7 x^{4}}\)
找到商:\(\dfrac{30 m^{5} n^{11}}{48 m^{10} n^{14}}\)
- 回答
-
\(\dfrac{5}{8 m^{5} n^{3}}\)
到目前为止,在所有示例中,在简化分数之前,没有在分子或分母方面做任何工作。 在下一个示例中,我们将首先在分子中找到两个单项式的乘积,然后再简化分数。 这遵循操作顺序。 请记住,分数条是一个分组符号。
找到商:\(\dfrac{\left(6 x^{2} y^{3}\right)\left(5 x^{3} y^{2}\right)}{\left(3 x^{4} y^{5}\right)}\)
- 回答
-
\(\begin{array} {lc} &\dfrac{\left(6 x^{2} y^{3}\right)\left(5 x^{3} y^{2}\right)}{\left(3 x^{4} y^{5}\right)}\\ \text { Simplify the numerator. }&\dfrac{30 x^{5} y^{5}}{3 x^{4} y^{5}} \\ \text { Simplify. } &10 x \end{array}\)
找到商:\(\dfrac{\left(6 a^{4} b^{5}\right)\left(4 a^{2} b^{5}\right)}{12 a^{5} b^{8}}\)
- 回答
-
\(2 a b^{2}\)
找到商:\(\dfrac{\left(-12 x^{6} y^{9}\right)\left(-4 x^{5} y^{8}\right)}{-12 x^{10} y^{12}}\)
- 回答
-
\(-4 x y^{5}\)
关键概念
- 指数的商属性:
- 如果 a 是实数,\(a\neq 0\)而 m, n 是整数,那么:\(\dfrac{a^{m}}{a^{n}}=a^{m-n}, m>n \text { and } \dfrac{a^{m}}{a^{n}}=\dfrac{1}{a^{m-n}}, n>m\)
- 零指数
- 如果 a 是非零数字,那么\(a^{0} =1\)。
- 指数@@ 的商与幂属性:
- 如果 a 和 b 是实数\(b\neq 0\),而 mm 是计数数,那么:\(\left(\dfrac{a}{b}\right)^{m}=\dfrac{a^{m}}{b^{m}}\)
- 要将分数提高到乘方,请将分子和分母提高到该次方。
- 指数属性摘要
- 如果 a, b 是实数而 m, nm, n 是整数,那么\(\begin{array}{lrll} \textbf{Product Property} & a^{m} \cdot a^{n} &=&a^{m+n} \\\textbf{Power Property} & \left(a^{m}\right)^{n} &=&a^{m \cdot n} \\\textbf{Product to a Power} & (a b)^{m} &=&a^{m} b^{m} \\ \textbf{Quotient Property} & \dfrac{a^{m}}{a^{n}} &=&a^{m-n}, a \neq 0, m>n \\ & \dfrac{a^{n}}{a^{n}} &=&1, a \neq 0, n>m \\\textbf{Zero Exponent Definition} &a^0&=&1,a\neq 0 \\\textbf{Quotient to a Power Property} & \left(\dfrac{a}{b}\right)^{m} &=&\dfrac{a^{m}}{b^{m}}, b \neq 0 \end{array}\)


