6.2: 求解绝对值方程

Last updated
Save as PDF

Page ID: 171266

Victoria Dominguez, Cristian Martinez, & Sanaa Saykali
Citrus College via ASCCC Open Educational Resources Initiative

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\dsum}{\displaystyle\sum\limits} $

$ \newcommand{\dint}{\displaystyle\int\limits} $

$ \newcommand{\dlim}{\displaystyle\lim\limits} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$\newcommand{\longvect}{\overrightarrow}$

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

要求解绝对值方程，首先要考虑绝对值的以下两个属性：

定义：绝对值的属性

属性 1：对于$b > 0$、$|a| = b$当且仅当$a = b$或$a = −b$

属性 2：适用于任何实数$a$$b$，$|a| = |b|$如果且仅当$a = b$或时$a = −b$

在应用属性 1 之前，将绝对值表达式隔离到方程的两侧。
通过将解替回原始方程来检查解。
解以解集的形式呈现$\{p, q\}$，其中$p$和$q$是任意实数。
绝对值方程的解集以数字线上的点的形式绘制。

示例 Template:Index

求解每个方程并绘制解集的图表。

$|x| = 7$
$|5x – 3| = 2$
$|20 – x| = −80$

解决方案

要求解$|x| = 7$，请使用带有 and$a = x$ 的属性 1$b = 7$。

因此，解是$x = 7$、$x = −7$和，解集是$\{-7,7\}$。解决方案集的图表如下图所示。

$clipboard_e4e661622afb19f733118b1049d678a57.png$

a 部分中使用的方程求解方法可以用$a = 5x – 3$和扩展到本部分中的给定方程$b = 2$。

因此，绝对值方程等同$|5x – 3| = 2$于：

$\begin{array} &&5x − 3 = 2 &\text{ or } &5x − 3 = −2 &\text{Property 1} \\ &5x = 5 &\text{ or } &5x = 1 &\text{Add \(3$到方程的两边}\\ &x = 1 &\ text {or} &x =\ dfrac {1} {5} &\ text {除以方程的$5$两边}\ end {array}\)

现在，检查给定绝对值方程的解是否$x = 1$和$x = \dfrac{1}{5}$解。

$\begin{array} &&\text{For } x = 1 &\text{For } x = \dfrac{1}{5} &\\ &|5x − 3| = 2 &|5x − 3| = 2 &\text{Given} \\ &|5(1) − 3| \stackrel{?}{=} 2 &|5 \left( \dfrac{1}{5} \right) − 3| \stackrel{?}{=} 2 &\text{Substitute the \(x$-values}\\ &|5 − 3|\ stackrel {?} {=} 2 &|1 − 3|\ stackrel {?} {=} 2 &\ text {Simplify}\\ &|2|\ stackrel {？} {=} 2 &|− 2|\ stackrel {?} {=} 2 &\ text {应用绝对值定义}\\ &2 = 2\;\ checkmark &2 = 2\;\ checkmark\ end {array}\)

既然上述方程是正确的，那么，$x = 1$并且$x = \dfrac{1}{5}$是给定绝对值方程的解。解决方案集是$\left\{\dfrac{1}{5} , 1\right\}$。解决方案集的图表如下图所示。

$clipboard_e78f4a9bbcf248134874230631b7dada3.png$

由于绝对值永远不能为负数，因此没有实数$x$可以$|20 – x| = −80$成立。方程没有解，解集为$∅$。

示例 Template:Index

求解并绘制解决方案集的图表。

$\left| \dfrac{4}{3} x + 3 \right| + 8 = 18$
$4 \left| \dfrac{1}{3}x − 6 \right| − 5 = −5$
$|4x – 3| = |x + 6|$

解决方案

请注意，绝对值表达式不是孤立的，这意味着无法应用这些属性。首先，在方程的左$\left| \dfrac{4}{3}x + 3 \right|$侧隔离，然后应用属性 1。

$\begin{array} &&\left| \dfrac{4}{3} x + 3 \right| + 8 = 18 &\text{Given equation} \\ & \left| \dfrac{4}{3} + 3 \right| = 10 &\text{Subtract \(8$从方程的两边}\ end {array}\)

现在隔离绝对值后，$\left| \dfrac{4}{3} + 3 \right| = 10$使用属性 1 求解，使用$a = \dfrac{4}{3} x + 3$和$b = 10$如下所示，

$\begin{array} && &\left| \dfrac{4}{3} + 3 \right| = 10 & & \\ &\dfrac{4}{3} + 3 = 10 &\text{ or } & \dfrac{4}{3} + 3 = -10 &\text{Property 1} \\ &\dfrac{4}{3} x = 7 &\text{ or } &\dfrac{4}{3}x = −13 &\text{Subtract \(3$来自两边}\\ &x =\ dfrac {21} {4} &\ text {or} &x = −\ dfrac {39} {4} &\ text {将两边乘以$\dfrac{3}{4}$}\ end {array}\)

检查解$x = −\dfrac{39}{4}$并将其$x = \dfrac{21}{4}$替换为原始绝对值方程。解决方案集为$\left\{ −\dfrac{39}{4}, \dfrac{21}{4} \right\}$，解决方案集的图形如下图所示。

$clipboard_e42b1bbc90f71c52e8a95664a185e2c67.png$

与 a 部分类似，隔离绝对值表达式。因此，首先$\left| \dfrac{1}{3} x − 6 \right|$在方程的左侧进行分离，然后应用属性 1。

$\begin{array} &&4 \left| \dfrac{1}{3}x − 6 \right| − 5 = −5 &\text{Given equation} \\ &4 \left| \dfrac{1}{3}x − 6 \right| = 0 &\text{Add \(5$到方程的两边}\\ &\ left|\ dfrac {1} {3} x − 6\ right| = 0 &\ text {除以方程的$4$两边}\ end {array}\)

绝对值是隔离的。由于$0$是唯一绝对值为的数字$0$，因此表达式$\dfrac{1}{3}x − 6$必须等于$0$。所以，

$\begin{array} &&\dfrac{1}{3}x − 6 = 0 & \\ &\dfrac{1}{3}x − 6 &\text{Add \(6$到方程的两边}\\ &x = 18 &\ text {将两边乘以$3$}\ end {array}\)

解决方案是$18$，解决方案集是$\{18\}$。验证它是否满足原始方程式。解决方案集的图表如下图所示。

$clipboard_e2acd5153df84bfef5569962926db627b.png$

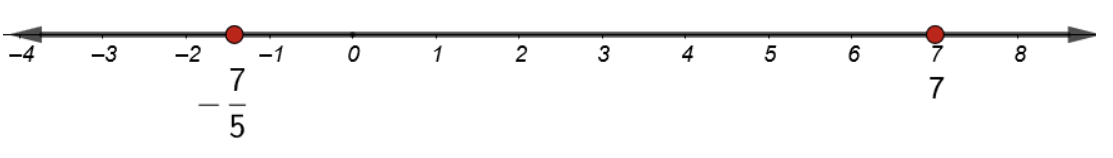
$|4x − 7| = |x + 14|$请注意，要求解$|4x − 7| = |x + 14|$，请使用带有$a = 4x − 7$和的属性 2$b = x + 14$。

$\begin{array} && &|4x − 7| = |x + 14| & &\text{Given} \\ &4x−7 = x+14 &\text{ or } &4x − 7 = −(x + 14) &\text{Property 2} \\ &4x−7 = x+14 &\text{ or } &4x − 7 = −x − 14 &\text{Distribute \(−1$为了简化右方程}\\ &4x = x + 21 &\ text {or} &4x = −x − 7 &\ text {$7$添加到每个等式的两边}\\ &3x = 21 &\ text {or} &5x = −7 &\ text {或} &x = −\ dfrac {7} {5} &\ text {将每个方程除以$x$-系数}\ end {array}\)

检查解$x = −\dfrac{7}{5}$并将其$x = 7$替换为原始绝对值方程。解决方案集是$\left\{ −\dfrac{7}{5}, 7\right\}$。解决方案的图表如下图所示。

$clipboard_eb0f0ab26e578678046e463bf1d8ac854.png$

练习 Template:Index

求解每个方程，检查解并绘制解集的图表。

$|x| = 19$
$|x − 4| = 10$
$|2x − 5| = 12$
$\left|\dfrac{x}{11} \right| = 2.5$
$|x − 3.8| = −2.7$
$|3x − 4.5| = 9.3$
$\dfrac{8}{3} |x − 6| = 14$
$|x + 15| − 19 = 7$
$|11x + 3| + 28 = 16$
$ \left| \dfrac{8}{7} x + 9 \right| − 2 = 8$
$ −3|2x − 7| + 13 = 13$
$ 8 − 5|10x + 6| = 5$
$ |5x − 14| = |3x − 9|$
$ |15x| = |x − 21|$
$ |4x − 7| = |5(2x + 3)|$
$ \dfrac{7}{8} = \dfrac{3x}{2} + \dfrac{2x}{5}$