4.11: 分段定义函数
- Last updated
- Oct 31, 2022
- Page ID
- 171162
- Save as PDF
分段定义函数是使用不同方程为域的不同部分定义的函数。
计算以下分段定义函数的给定值x，然后绘制函数的图形：
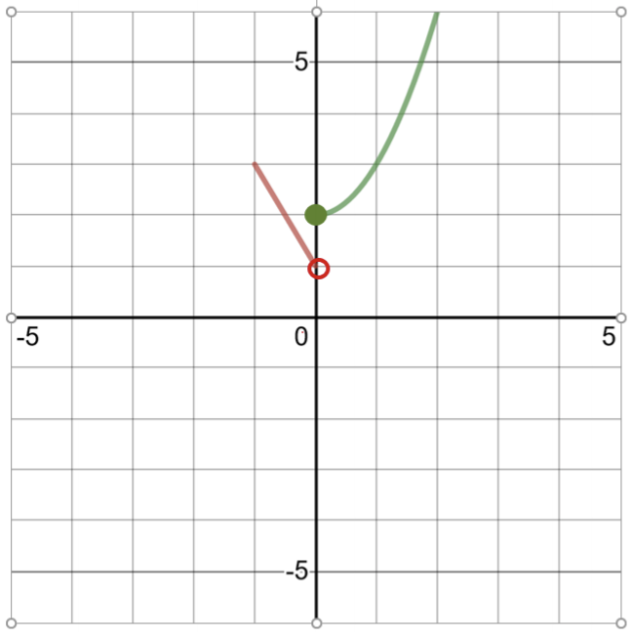
f(x)={−2x+1−1≤x<0x2+20≤x≤2
解决方案
要绘制此函数的图表，请制作解决方案表：
|
的解决方案表f(x)=−2x+1 域名−1≤x<0 |
|
| x | f(x) |
| -1 | 3 |
| 0 | 1（在此处打开圆圈，0 不在域中） |
|
的解决方案表f(x)=x2+2 域名0≤x≤2 |
|
| x | f(x) |
| 0 | 2 |
| 1 | 3 |
| 2 | 6 |
计算以下分段定义函数的给定值x，然后绘制函数的图形：
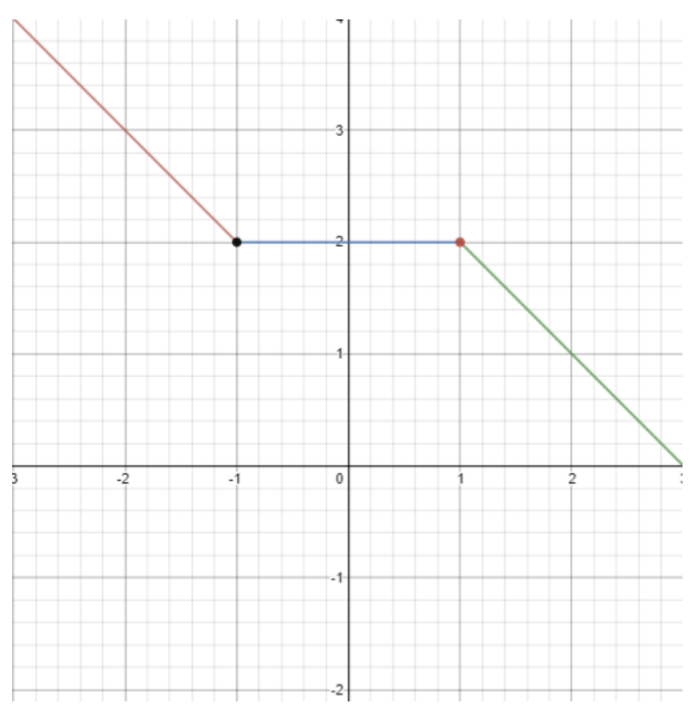
f(x)={−x+1x≤−12−1<x≤1−x+3x>1
解决方案
要绘制此函数的图表，请再次绘制解表：
|
的解决方案表f(x)=−x+1 域名x≤−1 |
|
| x | f(x) |
| -3 | 4 |
| -2 | 3 |
| -1 | 2（此处为封闭圆圈，域中为 -1） |
|
的解决方案表f(x)=2 域名−1<x≤1 |
|
| x | f(x) |
| -1 | 2（打开由上一个函数填充的圆圈，-1 不在域中） |
| 0 | 2 |
| 1 | 2（此处为闭圈，1 在域中） |
|
的解决方案表f(x)=−x+3 域名x>1 |
|
| x | f(x) |
| 1 | 2（打开由上一个函数填充的圆圈，1 不在域中） |
| 2 | 1 |
| 3 | 0 |
根据 x 的给定值计算以下分段定义的函数，然后绘制函数的图形：。
- \ (f (x) =\ 左\ {\ begin {array} {cc}
x & x<0\\
2 x+1 &x\ geq 0
\ end {array}\ 右。\) - g(x)={4−xx<22x−2x≥2
- h(x)={−x−1x<−10−1≤x≤1x+1x>1
- g(x)={6−8≤x<−43−4≤x≤5
- f(x)={−x+1−1≤x<1√x−11≤x≤5