Skip to main content
Global

9.9E：练习

  1. Last updated
  2. Save as PDF
  • Page ID
    204078

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    练习成就完美

    练习\(\PageIndex{11}\) Solve Quadratic Inequalities Graphically

    在以下练习中，

    1. 用图形求解
    2. 用间隔表示法写出解
      1. \(x^{2}+6 x+5>0\)
      2. \(x^{2}+4 x-12<0\)
      3. \(x^{2}+4 x+3 \leq 0\)
      4. \(x^{2}-6 x+8 \geq 0\)
      5. \(-x^{2}-3 x+18 \leq 0\)
      6. \(-x^{2}+2 x+24<0\)
      7. \(-x^{2}+x+12 \geq 0\)
      8. \(-x^{2}+2 x+15>0\)
    回答

    1。


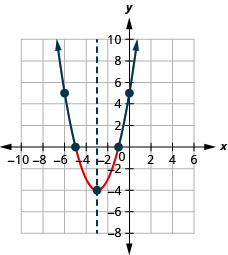
    1. 显示的图形是一个朝上的抛物线，具有顶点（负 3，负 4）和 y 截距（0,5）。
      图 9.8.16
    2. \((-\infty,-5) \cup(-1, \infty)\)

    3。


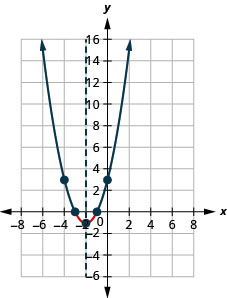
    1. 所示图形是一个朝上的抛物线，具有顶点（负 2，负 1）和 y 截距（0,3）。
      图 9.8.17
    2. \([-3,-1]\)

    5。


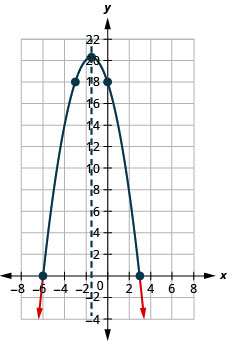
    1. 显示的图形是一个朝下的抛物线，其顶点（负十分之一 1 和 5，20）和 y 截距（0、18）。
      图 9.8.18
    2. \((-\infty,-6] \cup[3, \infty)\)

    7。


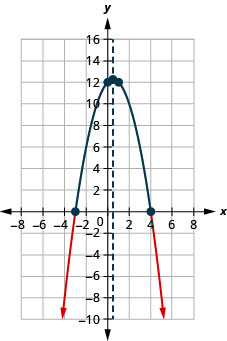
    1. 显示的图形是朝下的抛物线，Y 截距为 (0, 12)，x 截距为 (负 3, 0) 和 (4, 0)。
      图 9.8.19
    2. \([-3,4]\)
    练习\(\PageIndex{12}\) Solve Quadratic Inequalities Graphically

    在以下练习中，用代数求解每个不等式，并用区间表示法写出任何解。

    1. \(x^{2}+3 x-4 \geq 0\)
    2. \(x^{2}+x-6 \leq 0\)
    3. \(x^{2}-7 x+10<0\)
    4. \(x^{2}-4 x+3>0\)
    5. \(x^{2}+8 x>-15\)
    6. \(x^{2}+8 x<-12\)
    7. \(x^{2}-4 x+2 \leq 0\)
    8. \(-x^{2}+8 x-11<0\)
    9. \(x^{2}-10 x>-19\)
    10. \(x^{2}+6 x<-3\)
    11. \(-6 x^{2}+19 x-10 \geq 0\)
    12. \(-3 x^{2}-4 x+4 \leq 0\)
    13. \(-2 x^{2}+7 x+4 \geq 0\)
    14. \(2 x^{2}+5 x-12>0\)
    15. \(x^{2}+3 x+5>0\)
    16. \(x^{2}-3 x+6 \leq 0\)
    17. \(-x^{2}+x-7>0\)
    18. \(-x^{2}-4 x-5<0\)
    19. \(-2 x^{2}+8 x-10<0\)
    20. \(-x^{2}+2 x-7 \geq 0\)
    回答

    1。 \((-\infty,-4] \cup[1, \infty)\)

    3。 \((2,5)\)

    5。 \((-\infty,-5) \cup(-3, \infty)\)

    7。 \([2-\sqrt{2}, 2+\sqrt{2}]\)

    9。 \((-\infty, 5-\sqrt{6}) \cup(5+\sqrt{6}, \infty)\)

    11。 \(\left(-\infty,-\frac{5}{2}\right] \cup\left[-\frac{2}{3}, \infty\right)\)

    13。 \(\left[-\frac{1}{2}, 4\right]\)

    15。 \((-\infty, \infty)\)

    17. 没有解决办法

    19。 \((-\infty, \infty)\)

    练习\(\PageIndex{13}\) Writing Exercises
    1. 解释临界点以及如何使用它们来代数求解二次不等式。
    2. 以图形\(x^{2}+2x≥8\)和代数方式求解。 你更喜欢哪种方法，为什么？
    3. 以图形方式描述求解二次不等式所需的步骤。
    4. 描述用代数求解二次不等式所需的步骤。
    回答

    1。 答案可能有所不同。

    3。 答案可能有所不同。

    自检

    a. 完成练习后，使用此清单评估您对本节目标的掌握程度。

    此图是一个列表，用于评估您对本节中介绍的概念的理解。 它有 4 列标有 “我能”、“自信地”、“有帮助”，但不是，我不明白™ 在下面，我可以用图形求解二次不等式，用代数求解二次不等式。 其他栏目留空，供您检查是否理解。
    图 9.8.20

    b. 在 1-10 分中，根据你在清单上的回答，你会如何评价你对本节的掌握程度？ 你怎么能改善这个？