1.3: Kazi za Trigonometric

Last updated
Save as PDF

Page ID: 178955

Edwin “Jed” Herman & Gilbert Strang
OpenStax

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Malengo ya kujifunza

Badilisha hatua za angle kati ya digrii na radians.
Tambua ufafanuzi wa triangular na mviringo wa kazi za msingi za trigonometric.
Andika utambulisho wa msingi wa trigonometric.
Tambua grafu na vipindi vya kazi za trigonometric.
Eleza mabadiliko ya grafu ya sine au cosine kutoka kwa usawa wa kazi.

Kazi za trigonometric hutumiwa kutengeneza matukio mengi, ikiwa ni pamoja na mawimbi ya sauti, vibrations ya masharti, kubadilisha umeme wa sasa, na mwendo wa pendulums. Kwa kweli, karibu yoyote ya kurudia, au mzunguko, mwendo unaweza kuonyeshwa na mchanganyiko wa kazi za trigonometric. Katika sehemu hii, tunafafanua kazi sita za msingi za trigonometric na kuangalia baadhi ya utambulisho kuu unaohusisha kazi hizi.

Kipimo cha Radian

Ili kutumia kazi za trigonometric, sisi kwanza lazima tuelewe jinsi ya kupima pembe. Ingawa tunaweza kutumia radians na digrii zote mbili, radians ni kipimo cha asili zaidi kwa sababu zinahusiana moja kwa moja na mduara wa kitengo, mduara wenye radius 1. Kipimo cha radian cha angle kinafafanuliwa kama ifuatavyo. Kutokana na angle$θ$, hebu$s$ iwe urefu wa arc sambamba kwenye mduara wa kitengo (Kielelezo$\PageIndex{1}$). Tunasema angle sambamba na arc ya urefu 1 ina kipimo cha radian 1.

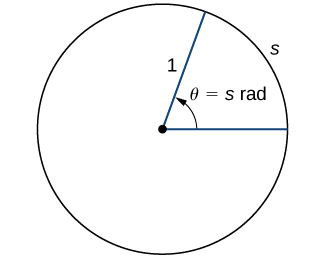
Picha ya mduara. Katika kituo halisi cha mduara kuna uhakika. Kutoka hatua hii, kuna sehemu moja ya mstari ambayo inaenea kwa usawa na haki hatua kwenye makali ya mduara na sehemu nyingine ya mstari ambayo inaenea diagonally kwenda juu na kulia kwa hatua nyingine kwenye makali ya mduara. Makundi haya ya mstari yana urefu wa kitengo 1. Sehemu ya mviringo kwenye makali ya mduara inayounganisha pointi mbili mwishoni mwa makundi ya mstari ni kinachoitwa “s”. Ndani ya mduara, kuna mshale unaoonyesha kutoka sehemu ya mstari usio na usawa hadi sehemu ya mstari wa diagonal. Mshale huu una lebo “theta = s radians”. — Kielelezo$\PageIndex{1}$: Kipimo cha radian cha angle$θ$ ni urefu wa arc$s$ ya arc inayohusishwa kwenye mduara wa kitengo.

Kwa kuwa angle ya$360°$ inalingana na mzunguko wa mduara, au arc ya urefu$2π$, tunahitimisha kuwa angle yenye kipimo cha shahada ya$360°$ ina kipimo cha radian cha$2π$. Vilevile, tunaona kwamba$180°$ ni sawa na$\pi$ radians. Jedwali$\PageIndex{1}$ linaonyesha uhusiano kati ya shahada ya kawaida na maadili ya radian.

Jedwali$\PageIndex{1}$: Angles za kawaida zilionyesha katika Degrees na Radians
Degrees	Radia	Degrees	Radia
0	0	120	$2π/3$
30	$π/6$	135	$3π/4$
45	$π/4$	150	$5π/6$
60	$π/3$	180	$π$
90	$π/2$

Kubadilisha kati ya Radians na Degrees

Express$225°$ using radians.
Express$5π/3$ rad using degrees.

Suluhisho

Tumia ukweli kwamba$180$° ni sawa na$\pi$ radians kama sababu ya uongofu (Jedwali$\PageIndex{1}$):

\[1=\dfrac{π \,\mathrm{rad}}{180°}=\dfrac{180°}{π \,\mathrm{rad}}. \nonumber \]

$225°=225°⋅\left(\dfrac{π}{180°}\right)=\left(\dfrac{5π}{4}\right)$ rad
$\dfrac{5π}{3}$ rad = $\dfrac{5π}{3}$⋅$\dfrac{180°}{π}$=$300$°

Zoezi$\PageIndex{1}$

Express$210°$ kutumia radians.
Express$11π/6$ rad using degrees.

Kidokezo: $π$radians ni sawa na 180°

Jibu

$7π/6$
330°

Kazi sita za Msingi za Trigonometric

Kazi za trigonometriki zinatuwezesha kutumia vipimo vya angle, kwa radians au digrii, ili kupata viwianishi vya uhakika kwenye mduara wowote-si tu kwenye mduara wa kitengo- au kupata angle iliyotolewa na uhakika kwenye mduara. Pia hufafanua uhusiano kati ya pande na pembe za pembetatu.

Ili kufafanua kazi za trigonometric, kwanza fikiria mduara wa kitengo unaozingatia asili na hatua$P=(x,y)$ kwenye mduara wa kitengo. Hebu$θ$ kuwa angle na upande wa awali ulio pamoja na$x$ mhimili mzuri na kwa upande wa terminal ambayo ni sehemu ya mstari$OP$. Pembe katika nafasi hii inasemekana kuwa katika nafasi ya kawaida (Kielelezo$\PageIndex{2}$). Tunaweza kisha kufafanua maadili ya kazi sita trigonometric kwa$θ$ katika suala la kuratibu$x$ na$y$.

Picha ya grafu. Grafu ina mduara uliopangwa juu yake, na katikati ya mduara katika asili, ambapo kuna uhakika. Kutoka hatua hii, kuna sehemu moja ya mstari ambayo inaenea kwa usawa pamoja na mhimili x na haki ya uhakika kwenye makali ya mduara. Kuna sehemu nyingine ya mstari inayoendelea diagonally hadi juu na kulia kwa hatua nyingine kwenye makali ya mduara. Hatua hii inaitwa “P = (x, y)”. Makundi haya ya mstari yana urefu wa kitengo 1. Kutoka hatua ya “P”, kuna mstari wa wima wa dotted unaoendelea chini mpaka unapiga mhimili wa x na hivyo sehemu ya mstari wa usawa. Ndani ya mduara, kuna mshale unaoonyesha kutoka sehemu ya mstari usio na usawa hadi sehemu ya mstari wa diagonal. Mshale huu una lebo “theta”. — Kielelezo$\PageIndex{2}$: Angle$θ$ iko katika nafasi ya kawaida. Maadili ya kazi za trigonometric kwa$θ$ hufafanuliwa kwa suala la kuratibu$x$ na$y$.

Ufafanuzi: Kazi za Trigonometric

Hebu$P=(x,y)$ kuwa hatua juu ya mduara kitengo unaozingatia katika asili$O$. Hebu$θ$ kuwa angle na upande wa kwanza pamoja na$x$ mhimili mzuri na upande wa terminal uliotolewa na sehemu ya mstari$OP$. Kazi ya trigonometric hufafanuliwa kama

$\sin θ=y$	$\csc θ=\dfrac{1}{y}$
$\cos θ=x$	$\sec θ=\dfrac{1}{x}$
$\tan θ=\dfrac{y}{x}$	$\cot θ=\dfrac{x}{y}$

Ikiwa$x=0, \sec θ$ na$\tan θ$ haijulikani. Ikiwa$y=0$, basi$\cot θ$ na$\csc θ$ haijulikani.

Tunaweza kuona kwamba kwa uhakika$P=(x,y)$ juu ya mduara wa radius$r$ na angle sambamba$θ$, kuratibu$x$ na$y$ kukidhi

\[\begin{align} \cos θ &=\dfrac{x}{r} \\ x &=r\cos θ \end{align} \nonumber \]

\[\begin{align} \sin θ &=\dfrac{y}{r} \\ y &=r\sin θ. \end{align} \nonumber \]

Maadili ya kazi nyingine za trigonometric zinaweza kuelezwa kwa suala la$x,y$, na$r$ (Kielelezo$\PageIndex{3}$).

Kielelezo$\PageIndex{3}$: Kwa uhakika$P=(x,y)$ juu ya mduara wa Radius$r$, kuratibu$x$ na$y$ kukidhi$x=r\cos θ$ na$y=r\sin θ$.

Jedwali$\PageIndex{2}$ linaonyesha maadili ya sine na cosine kwenye pembe kuu katika quadrant ya kwanza. Kutoka meza hii, tunaweza kuamua maadili ya sine na cosine kwenye pembe zinazofanana katika quadrants nyingine. Maadili ya kazi nyingine za trigonometric huhesabiwa kwa urahisi kutoka kwa maadili ya$\sin θ$ and $\cos θ.$

Jedwali$\PageIndex{2}$: Maadili ya$\sin θ$ na$θ$ katika$\cos θ$ pembe kuu katika Quadrant ya Kwanza
$θ$	$\sin θ$	$\cos θ$
\ (ε\) ε” style="text-align:katikati; "> 0	\ (\ sin 合\)” style="Nakala-align:center; "> 0	\ (\ cos 合\)” style="Nakala-align:katikati; "> 1
\ (合\) 合” style="text-align:katikati; ">$\dfrac{π}{6}$	\ (\ sin 合\)” style="text-align:center; ">$\dfrac{1}{2}$	\ (\ cos 合\)” style="Nakala-align:center; ">$\dfrac{\sqrt{3}}{2}$
\ (合\) 合” style="text-align:katikati; ">$\dfrac{π}{4}$	\ (\ sin 合\)” style="text-align:center; ">$\dfrac{\sqrt{2}}{2}$	\ (\ cos 合\)” style="Nakala-align:center; ">$\dfrac{\sqrt{2}}{2}$
\ (合\) 合” style="text-align:katikati; ">$\dfrac{π}{3}$	\ (\ sin 合\)” style="text-align:center; ">$\dfrac{\sqrt{3}}{2}$	\ (\ cos 合\)” style="Nakala-align:center; ">$\dfrac{1}{2}$
\ (合\) 合” style="text-align:katikati; ">$\dfrac{π}{2}$	\ (\ sin 合\)” style="text-align:katikati; "> 1	\ (\ cos 合\)” style="Nakala-align:katikati; "> 0

Mfano$\PageIndex{2}$: Evaluating Trigonometric Functions

Tathmini kila moja ya maneno yafuatayo.

$\sin \left(\dfrac{2π}{3} \right)$
$\cos \left(−\dfrac{5π}{6} \right)$
$\tan \left(\dfrac{15π}{4}\right)$

Suluhisho:

a) Kwenye mduara wa kitengo, angle$θ=\dfrac{2π}{3}$ inafanana na uhakika$\left(−\dfrac{1}{2},\dfrac{\sqrt{3}}{2}\right)$. Kwa hiyo,

\[ \sin \left(\dfrac{2π}{3}\right)=y=\left(\dfrac{\sqrt{3}}{2}\right). \nonumber \]

$Picha ya grafu. Grafu ina mduara uliopangwa juu yake, na katikati ya mduara katika asili, ambapo kuna uhakika. Kutoka hatua hii, kuna sehemu moja ya mstari ambayo inaenea kwa usawa pamoja na mhimili x na haki ya uhakika kwenye makali ya mduara. Kuna sehemu nyingine ya mstari inayoendelea diagonally hadi juu na kushoto hadi hatua nyingine kwenye makali ya mduara. Hatua hii inaitwa “(- (1/2), ((mizizi ya mraba ya 3) /2)”. Makundi haya ya mstari yana urefu wa kitengo 1. Kutoka hatua “(- (1/2), ((mizizi ya mraba ya 3) /2)”, kuna mstari wa wima unaoendelea chini mpaka unapiga mhimili wa x. Ndani ya mduara, kuna mshale wa pembe unaoanza kwenye sehemu ya mstari usio na usawa na husafiri kinyume mpaka unapopiga sehemu ya mstari wa diagonal. Mshale huu una lebo “theta = (2 pi) /3”.$

b) Angle$θ=−\dfrac{5π}{6}$ corresponds to a revolution in the negative direction, as shown. Therefore,

\[\cos \left(−\dfrac{5π}{6}\right)=x=−\dfrac{\sqrt{3}}{2}. \nonumber \]

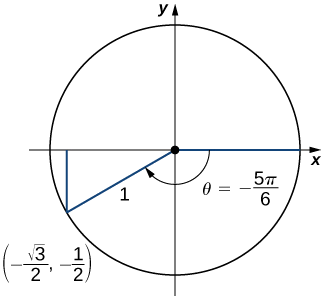
$An image of a graph. The graph has a circle plotted on it, with the center of the circle at the origin, where there is a point. From this point, there is one line segment that extends horizontally along the x axis to the right to a point on the edge of the circle. There is another line segment that extends diagonally downwards and to the left to another point on the edge of the circle. This point is labeled “(-((square root of 3)/2)), -(1/2))”. These line segments have a length of 1 unit. From the point “(-((square root of 3)/2)), -(1/2))”, there is a vertical line that extends upwards until it hits the x axis. Inside the circle, there is a curved arrow that starts at the horizontal line segment and travels clockwise until it hits the diagonal line segment. This arrow has the label “theta = -(5 pi)/6”.$

c) An angle $θ$=$\dfrac{15π}{4}$=$2π$+$\dfrac{7π}{4}$. Therefore, this angle corresponds to more than one revolution, as shown. Knowing the fact that an angle of $\dfrac{7π}{4}$ corresponds to the point $(\dfrac{\sqrt{2}}{2},-\dfrac{\sqrt{2}}{2})$, we can conclude that

\[\tan \left(\dfrac{15π}{4}\right)=\dfrac{y}{x}=−1. \nonumber \]

$An image of a graph. The graph has a circle plotted on it, with the center of the circle at the origin, where there is a point. From this point, there is one line segment that extends horizontally along the x axis to the right to a point on the edge of the circle. There is another line segment that extends diagonally downwards and to the right to another point on the edge of the circle. This point is labeled “(((square root of 2)/2), -((square root of 2)/2))”. These line segments have a length of 1 unit. From the point “(((square root of 2)/2), -((square root of 2)/2))”, there is a vertical line that extends upwards until it hits the x axis and thus the horizontal line segment. Inside the circle, there is a curved arrow that starts at the horizontal line segment and travels counterclockwise. The arrow makes one full rotation around the circle and then keeps traveling until it hits the diagonal line segment. This arrow has the label “theta = (15 pi)/4”.$

Exercise $\PageIndex{2}$

Evaluate $\cos(3π/4)$ and $\sin(−π/6)$.

Hint: Look at angles on the unit circle.

Answer

\[\cos(3π/4) = −\sqrt{2}/2\nonumber \]

\[ \sin(−π/6) =−1/2 \nonumber \]

As mentioned earlier, the ratios of the side lengths of a right triangle can be expressed in terms of the trigonometric functions evaluated at either of the acute angles of the triangle. Let $θ$ be one of the acute angles. Let $A$ be the length of the adjacent leg, $O$ be the length of the opposite leg, and $H$ be the length of the hypotenuse. By inscribing the triangle into a circle of radius $H$, as shown in Figure $\PageIndex{4}$, we see that $A,H$, and $O$ satisfy the following relationships with $θ$:

$\sin θ=\dfrac{O}{H}$	$\csc θ=\dfrac{H}{O}$
$\cos θ=\dfrac{A}{H}$	$\sec θ=\dfrac{H}{A}$
$\tan θ=\dfrac{O}{A}$	$\cot θ=\dfrac{A}{O}$

An image of a graph. The graph has a circle plotted on it, with the center of the circle at the origin, where there is a point. From this point, there is one line segment that extends horizontally along the x axis to the right to a point on the edge of the circle. There is another line segment with length labeled “H” that extends diagonally upwards and to the right to another point on the edge of the circle. From the point, there is vertical line with a length labeled “O” that extends downwards until it hits the x axis and thus the horizontal line segment at a point with a right triangle symbol. The distance from this point to the center of the circle is labeled “A”. Inside the circle, there is an arrow that points from the horizontal line segment to the diagonal line segment. This arrow has the label “theta”. — Figure $\PageIndex{4}$: By inscribing a right triangle in a circle, we can express the ratios of the side lengths in terms of the trigonometric functions evaluated at $θ$.

Example $\PageIndex{3}$: Constructing a Wooden Ramp

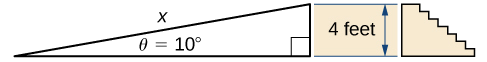
A wooden ramp is to be built with one end on the ground and the other end at the top of a short staircase. If the top of the staircase is $4$ ft from the ground and the angle between the ground and the ramp is to be $10$°, how long does the ramp need to be?

Solution

Let $x$ denote the length of the ramp. In the following image, we see that $x$ needs to satisfy the equation $\sin(10°)=4/x$. Solving this equation for $x$, we see that $x=4/\sin(10°)$≈$23.035$ ft.

$An image of a ramp and a staircase. The ramp starts at a point and increases diagonally upwards and to the right at an angle of 10 degrees for x feet. At the end of the ramp, which is 4 feet off the ground, a staircase descends downwards and to the right.$

Exercise $\PageIndex{3}$

A house painter wants to lean a $20$-ft ladder against a house. If the angle between the base of the ladder and the ground is to be $60$°, how far from the house should she place the base of the ladder?

Hint: Draw a right triangle with hypotenuse 20.

Answer: 10 ft

Trigonometric Identities

A trigonometric identity is an equation involving trigonometric functions that is true for all angles $θ$ for which the functions are defined. We can use the identities to help us solve or simplify equations. The main trigonometric identities are listed next.

Trigonometric Identities

Reciprocal identities

\[\tan θ=\dfrac{\sin θ}{\cos θ} \nonumber \]

\[\cot θ=\dfrac{\cos θ}{\sin θ} \nonumber \]

\[\csc θ=\dfrac{1}{\sin θ} \nonumber \]

\[\sec θ=\dfrac{1}{\cos θ} \nonumber \]

Pythagorean identities

\[\begin{align} \sin^2θ+\cos^2θ &=1 \label{py1} \\[4pt] 1+\tan^2θ &=\sec^2θ \\[4pt] 1+\cot^2θ &=\csc^2θ \end{align} \]

Addition and subtraction formulas

\[\sin(α±β)=\sin α\cos β±\cos α \sin β \nonumber \]

\[\cos(α±β)=\cos α\cos β∓\sin α \sin β \nonumber \]

Double-angle formulas

\[\sin(2θ)=2\sin θ\cos θ \label{double1} \]

\[\begin{align} \cos(2θ) &=2\cos^2θ−1 \\[4pt] &=1−2\sin^2θ \\[4pt] &=\cos^2θ−\sin^2θ \end{align} \nonumber \]

Example $\PageIndex{4}$: Solving Trigonometric Equations

For each of the following equations, use a trigonometric identity to find all solutions.

$1+\cos(2θ)=\cos θ$
$\sin(2θ)=\tan θ$

Solution

a) Using the double-angle formula for $\cos(2θ)$, we see that $θ$ is a solution of

\[1+\cos(2θ)=\cos θ \nonumber \]

if and only if

\[1+2\cos^2θ−1=\cos θ, \nonumber \]

which is true if and only if

\[2\cos^2θ−\cos θ=0. \nonumber \]

To solve this equation, it is important to note that we need to factor the left-hand side and not divide both sides of the equation by $\cos θ$. The problem with dividing by $\cos θ$ is that it is possible that $\cos θ$ is zero. In fact, if we did divide both sides of the equation by $\cos θ$, we would miss some of the solutions of the original equation. Factoring the left-hand side of the equation, we see that $θ$ is a solution of this equation if and only if

\[\cos θ(2\cos θ−1)=0. \nonumber \]

Since $\cos θ=0$ when

\[θ=\dfrac{π}{2},\dfrac{π}{2}±π,\dfrac{π}{2}±2π,…, \nonumber \]

and $\cos θ=1/2$ when

\[θ=\dfrac{π}{3},\dfrac{π}{3}±2π,…\mathrm{or}\ θ=−\dfrac{π}{3},−\dfrac{π}{3}±2π,…, \nonumber \]

we conclude that the set of solutions to this equation is

\[θ=\dfrac{π}{2}+nπ,\;θ=\dfrac{π}{3}+2nπ \nonumber \]

and

\[θ=−\dfrac{π}{3}+2nπ,\;n=0,±1,±2,….\nonumber \]

b) Using the double-angle formula for $\sin(2θ)$ and the reciprocal identity for $\tan(θ)$, the equation can be written as

\[2\sin θ\cos θ=\dfrac{\sin θ}{\cos θ}.\nonumber \]

To solve this equation, we multiply both sides by $\cos θ$ to eliminate the denominator, and say that if $θ$ satisfies this equation, then $θ$ satisfies the equation

\[2\sin θ \cos^2θ−\sin θ=0. \nonumber \]

However, we need to be a little careful here. Even if $θ$ satisfies this new equation, it may not satisfy the original equation because, to satisfy the original equation, we would need to be able to divide both sides of the equation by $\cos θ$. However, if $\cos θ=0$, we cannot divide both sides of the equation by $\cos θ$. Therefore, it is possible that we may arrive at extraneous solutions. So, at the end, it is important to check for extraneous solutions. Returning to the equation, it is important that we factor $\sin θ$ out of both terms on the left-hand side instead of dividing both sides of the equation by $\sin θ$. Factoring the left-hand side of the equation, we can rewrite this equation as

\[\sin θ(2\cos^2θ−1)=0. \nonumber \]

Therefore, the solutions are given by the angles $θ$ such that $\sin θ=0$ or $\cos^2θ=1/2$. The solutions of the first equation are $θ=0,±π,±2π,….$ The solutions of the second equation are $θ=π/4,(π/4)±(π/2),(π/4)±π,….$ After checking for extraneous solutions, the set of solutions to the equation is

\[θ=nπ \nonumber \]

and

\[θ=\dfrac{π}{4}+\dfrac{nπ}{2} \nonumber \]

with $n=0,±1,±2,….$

Exercise $\PageIndex{4}$

Find all solutions to the equation $\cos(2θ)=\sin θ.$

Hint: Use the double-angle formula for cosine (Equation \ref{double1}).

Answer

$θ=\dfrac{3π}{2}+2nπ,\dfrac{π}{6}+2nπ,\dfrac{5π}{6}+2nπ$

for $n=0,±1,±2,…$.

Example $\PageIndex{5}$: Proving a Trigonometric Identity

Prove the trigonometric identity $1+\tan^2θ=\sec^2θ.$

Solution:

We start with the Pythagorean identity (Equation \ref{py1})

\[\sin^2θ+\cos^2θ=1. \nonumber \]

Dividing both sides of this equation by $\cos^2θ,$ we obtain

\[\dfrac{\sin^2θ}{\cos^2θ}+1=\dfrac{1}{\cos^2θ}. \nonumber \]

Since $\sin θ/\cos θ=\tan θ$ and $1/\cos θ=\sec θ$, we conclude that

\[\tan^2θ+1=\sec^2θ. \nonumber \]

Exercise $\PageIndex{5}$

Prove the trigonometric identity $1+\cot^2θ=\csc^2θ.$

Answer: Divide both sides of the identity $\sin^2θ+\cos^2θ=1$ by $\sin^2θ$.

Graphs and Periods of the Trigonometric Functions

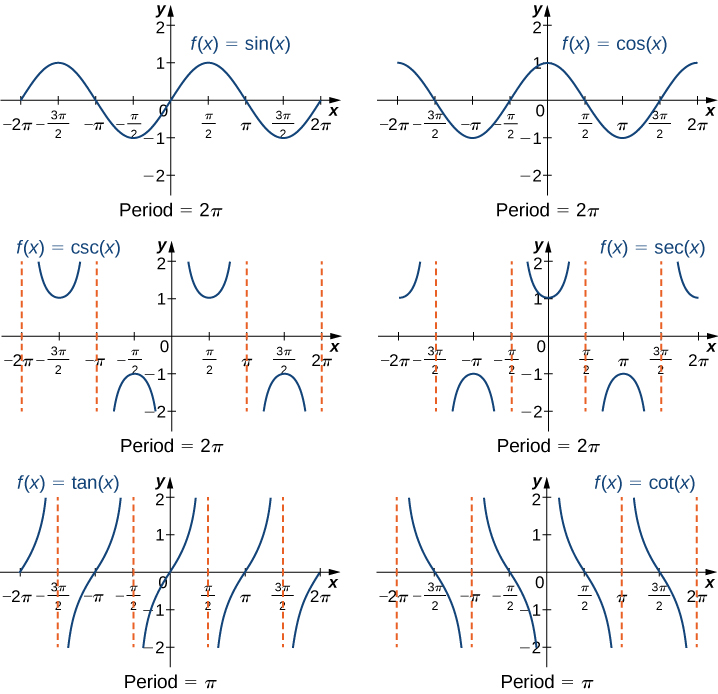
We have seen that as we travel around the unit circle, the values of the trigonometric functions repeat. We can see this pattern in the graphs of the functions. Let $P=(x,y)$ be a point on the unit circle and let $θ$ be the corresponding angle . Since the angle $θ$ and $θ+2π$ correspond to the same point $P$, the values of the trigonometric functions at $θ$ and at $θ+2π$ are the same. Consequently, the trigonometric functions are periodic functions. The period of a function $f$ is defined to be the smallest positive value $p$ such that $f(x+p)=f(x)$ for all values $x$ in the domain of $f$. The sine, cosine, secant, and cosecant functions have a period of $2π$. Since the tangent and cotangent functions repeat on an interval of length $π$, their period is $π$ (Figure $\PageIndex{5}$).

An image of six graphs. Each graph has an x axis that runs from -2 pi to 2 pi and a y axis that runs from -2 to 2. The first graph is of the function “f(x) = sin(x)”, which is a curved wave function. The graph of the function starts at the point (-2 pi, 0) and increases until the point (-((3 pi)/2), 1). After this point, the function decreases until the point (-(pi/2), -1). After this point, the function increases until the point ((pi/2), 1). After this point, the function decreases until the point (((3 pi)/2), -1). After this point, the function begins to increase again. The x intercepts shown on the graph are at the points (-2 pi, 0), (-pi, 0), (0, 0), (pi, 0), and (2 pi, 0). The y intercept is at the origin. The second graph is of the function “f(x) = cos(x)”, which is a curved wave function. The graph of the function starts at the point (-2 pi, 1) and decreases until the point (-pi, -1). After this point, the function increases until the point (0, 1). After this point, the function decreases until the point (pi, -1). After this point, the function increases again. The x intercepts shown on the graph are at the points (-((3 pi)/2), 0), (-(pi/2), 0), ((pi/2), 0), and (((3 pi)/2), 0). The y intercept is at the point (0, 1). The graph of cos(x) is the same as the graph of sin(x), except it is shifted to the left by a distance of (pi/2). On the next four graphs there are dotted vertical lines which are not a part of the function, but act as boundaries for the function, boundaries the function will never touch. They are known as vertical asymptotes. There are infinite vertical asymptotes for all of these functions, but these graphs only show a few. The third graph is of the function “f(x) = csc(x)”. The vertical asymptotes for “f(x) = csc(x)” on this graph occur at “x = -2 pi”, “x = -pi”, “x = 0”, “x = pi”, and “x = 2 pi”. Between the “x = -2 pi” and “x = -pi” asymptotes, the function looks like an upward facing “U”, with a minimum at the point (-((3 pi)/2), 1). Between the “x = -pi” and “x = 0” asymptotes, the function looks like an downward facing “U”, with a maximum at the point (-(pi/2), -1). Between the “x = 0” and “x = pi” asymptotes, the function looks like an upward facing “U”, with a minimum at the point ((pi/2), 1). Between the “x = pi” and “x = 2 pi” asymptotes, the function looks like an downward facing “U”, with a maximum at the point (((3 pi)/2), -1). The fourth graph is of the function “f(x) = sec(x)”. The vertical asymptotes for this function on this graph are at “x = -((3 pi)/2)”, “x = -(pi/2)”, “x = (pi/2)”, and “x = ((3 pi)/2)”. Between the “x = -((3 pi)/2)” and “x = -(pi/2)” asymptotes, the function looks like an downward facing “U”, with a maximum at the point (-pi, -1). Between the “x = -(pi/2)” and “x = (pi/2)” asymptotes, the function looks like an upward facing “U”, with a minimum at the point (0, 1). Between the “x = (pi/2)” and “x = (3pi/2)” asymptotes, the function looks like an downward facing “U”, with a maximum at the point (pi, -1). The graph of sec(x) is the same as the graph of csc(x), except it is shifted to the left by a distance of (pi/2). The fifth graph is of the function “f(x) = tan(x)”. The vertical asymptotes of this function on this graph occur at “x = -((3 pi)/2)”, “x = -(pi/2)”, “x = (pi/2)”, and “x = ((3 pi)/2)”. In between all of the vertical asymptotes, the function is always increasing but it never touches the asymptotes. The x intercepts on this graph occur at the points (-2 pi, 0), (-pi, 0), (0, 0), (pi, 0), and (2 pi, 0). The y intercept is at the origin. The sixth graph is of the function “f(x) = cot(x)”. The vertical asymptotes of this function on this graph occur at “x = -2 pi”, “x = -pi”, “x = 0”, “x = pi”, and “x = 2 pi”. In between all of the vertical asymptotes, the function is always decreasing but it never touches the asymptotes. The x intercepts on this graph occur at the points (-((3 pi)/2), 0), (-(pi/2), 0), ((pi/2), 0), and (((3 pi)/2), 0) and there is no y intercept. — Figure $\PageIndex{5}$: The six trigonometric functions are periodic.

Just as with algebraic functions, we can apply transformations to trigonometric functions. In particular, consider the following function:

\[f(x)=A\sin(B(x−α))+C. \nonumber \]

In Figure $\PageIndex{6}$, the constant $α$ causes a horizontal or phase shift. The factor $B$ changes the period. This transformed sine function will have a period $2π/|B|$. The factor $A$ results in a vertical stretch by a factor of $|A|$. We say $|A|$ is the “amplitude of $f$.” The constant $C$ causes a vertical shift.

An image of a graph. The graph is of the function “f(x) = Asin(B(x - alpha)) + C”. Along the y axis, there are 3 hash marks: starting from the bottom and moving up, the hash marks are at the values “C - A”, “C”, and “C + A”. The distance from the origin to “C” is labeled “vertical shift”. The distance from “C - A” to “A” and the distance from “A” to “C + A” is “A”, which is labeled “amplitude”. On the x axis is a hash mark at the value “alpha” and the distance between the origin and “alpha” is labeled “horizontal shift”. The distance between two successive minimum values of the function (in other words, the distance between two bottom parts of the wave that are next to each other) is “(2 pi)/(absolute value of B)” is labeled the period. The period is also the distance between two successive maximum values of the function. — Figure $\PageIndex{6}$: A graph of a general sine function.

Notice in Figure $\PageIndex{6}$ that the graph of $y=\cos x$ is the graph of $y=\sin x$ shifted to the left $π/2$ units. Therefore, we can write

\[\cos x=\sin(x+π/2). \nonumber \]

Similarly, we can view the graph of $y=\sin x$ as the graph of $y=\cos x$ shifted right $π/2$ units, and state that $\sin x=\cos(x−π/2).$

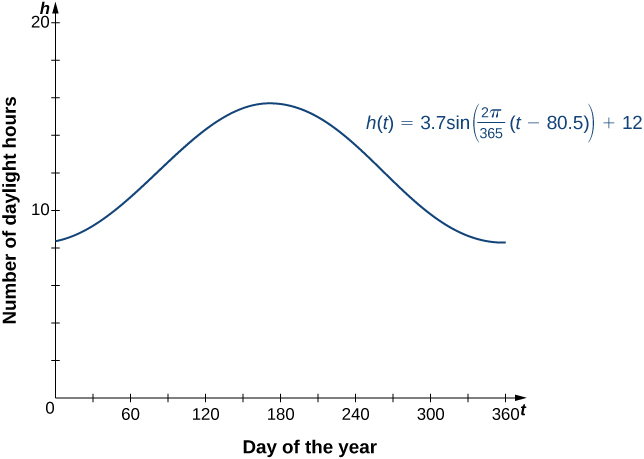
A shifted sine curve arises naturally when graphing the number of hours of daylight in a given location as a function of the day of the year. For example, suppose a city reports that June 21 is the longest day of the year with 15.7 hours and December 21 is the shortest day of the year with 8.3 hours. It can be shown that the function

\[h(t)=3.7\sin \left(\dfrac{2π}{365}(x−80.5)\right)+12 \nonumber \]

is a model for the number of hours of daylight $h$ as a function of day of the year $t$ (Figure $\PageIndex{7}$).

An image of a graph. The x axis runs from 0 to 365 and is labeled “t, day of the year”. The y axis runs from 0 to 20 and is labeled “h, number of daylight hours”. The graph is of the function “h(t) = 3.7sin(((2 pi)/365)(t - 80.5)) + 12”, which is a curved wave function. The function starts at the approximate point (0, 8.4) and begins increasing until the approximate point (171.8, 15.7). After this point, the function decreases until the approximate point (354.3, 8.3). After this point, the function begins increasing again. — Figure $\PageIndex{7}$: The hours of daylight as a function of day of the year can be modeled by a shifted sine curve.

Example $\PageIndex{6}$: Sketching the Graph of a Transformed Sine Curve

Sketch a graph of $f(x)=3\sin(2(x−\frac{π}{4}))+1.$

Solution

This graph is a phase shift of $y=\sin (x)$ to the right by $π/4$ units, followed by a horizontal compression by a factor of 2, a vertical stretch by a factor of 3, and then a vertical shift by 1 unit. The period of $f$ is $π$.

$An image of a graph. The x axis runs from -((3 pi)/2) to 2 pi and the y axis runs from -3 to 5. The graph is of the function “f(x) = 3sin(2(x-(pi/4))) + 1”, which is a curved wave function. The function starts decreasing from the point (-((3 pi)/2), 4) until it hits the point (-pi, -2). At this point, the function begins increasing until it hits the point (-(pi/2), 4). After this point, the function begins decreasing until it hits the point (0, -2). After this point, the function increases until it hits the point ((pi/2), 4). After this point, the function decreases until it hits the point (pi, -2). After this point, the function increases until it hits the point (((3 pi)/2), 4). After this point, the function decreases again.$

Exercise $\PageIndex{6}$

Describe the relationship between the graph of $f(x)=3\sin(4x)−5$ and the graph of $y=\sin(x)$.

Hint: The graph of $f$ can be sketched using the graph of $y=\sin(x)$ and a sequence of three transformations.

Answer: To graph $f(x)=3\sin(4x)−5$, the graph of $y=\sin(x)$ needs to be compressed horizontally by a factor of 4, then stretched vertically by a factor of 3, then shifted down 5 units. The function $f$ will have a period of $π/2$ and an amplitude of 3.

Key Concepts

Radian measure is defined such that the angle associated with the arc of length 1 on the unit circle has radian measure 1. An angle with a degree measure of $180$° has a radian measure of $\pi$ rad.
For acute angles $θ$,the values of the trigonometric functions are defined as ratios of two sides of a right triangle in which one of the acute angles is $θ$.
For a general angle $θ$, let $(x,y)$ be a point on a circle of radius $r$ corresponding to this angle $θ$. The trigonometric functions can be written as ratios involving $x$, $y$, and $r$.
The trigonometric functions are periodic. The sine, cosine, secant, and cosecant functions have period $2π$. The tangent and cotangent functions have period $π$.

Key Equations

Generalized sine function

$f(x)=A \sin(B(x−α))+C$

Glossary

periodic function: a function is periodic if it has a repeating pattern as the values of $x$ move from left to right

radians: for a circular arc of length $s$ on a circle of radius 1, the radian measure of the associated angle $θ$ is $s$

trigonometric functions: functions of an angle defined as ratios of the lengths of the sides of a right triangle

trigonometric identity: an equation involving trigonometric functions that is true for all angles $θ$ for which the functions in the equation are defined

\(\sin θ=y\)	\(\csc θ=\dfrac{1}{y}\)
\(\cos θ=x\)	\(\sec θ=\dfrac{1}{x}\)
\(\tan θ=\dfrac{y}{x}\)	\(\cot θ=\dfrac{x}{y}\)

\(θ\)	\(\sin θ\)	\(\cos θ\)
\ (ε\) ε” style="text-align:katikati; "> 0	\ (\ sin 合\)” style="Nakala-align:center; "> 0	\ (\ cos 合\)” style="Nakala-align:katikati; "> 1
\ (合\) 合” style="text-align:katikati; ">\(\dfrac{π}{6}\)	\ (\ sin 合\)” style="text-align:center; ">\(\dfrac{1}{2}\)	\ (\ cos 合\)” style="Nakala-align:center; ">\(\dfrac{\sqrt{3}}{2}\)
\ (合\) 合” style="text-align:katikati; ">\(\dfrac{π}{4}\)	\ (\ sin 合\)” style="text-align:center; ">\(\dfrac{\sqrt{2}}{2}\)	\ (\ cos 合\)” style="Nakala-align:center; ">\(\dfrac{\sqrt{2}}{2}\)
\ (合\) 合” style="text-align:katikati; ">\(\dfrac{π}{3}\)	\ (\ sin 合\)” style="text-align:center; ">\(\dfrac{\sqrt{3}}{2}\)	\ (\ cos 合\)” style="Nakala-align:center; ">\(\dfrac{1}{2}\)
\ (合\) 合” style="text-align:katikati; ">\(\dfrac{π}{2}\)	\ (\ sin 合\)” style="text-align:katikati; "> 1	\ (\ cos 合\)” style="Nakala-align:katikati; "> 0

\(\sin θ=\dfrac{O}{H}\)	\(\csc θ=\dfrac{H}{O}\)
\(\cos θ=\dfrac{A}{H}\)	\(\sec θ=\dfrac{H}{A}\)
\(\tan θ=\dfrac{O}{A}\)	\(\cot θ=\dfrac{A}{O}\)

Kubadilisha kati ya Radians na Degrees

Zoezi\(\PageIndex{1}\)

Ufafanuzi: Kazi za Trigonometric

Mfano\(\PageIndex{2}\): Evaluating Trigonometric Functions

Exercise \(\PageIndex{2}\)

Example \(\PageIndex{3}\): Constructing a Wooden Ramp

Exercise \(\PageIndex{3}\)

Trigonometric Identities

Example \(\PageIndex{4}\): Solving Trigonometric Equations

Exercise \(\PageIndex{4}\)

Example \(\PageIndex{5}\): Proving a Trigonometric Identity

Exercise \(\PageIndex{5}\)

Example \(\PageIndex{6}\): Sketching the Graph of a Transformed Sine Curve

Exercise \(\PageIndex{6}\)

Degrees	Radia	Degrees	Radia
0	0	120	\(2π/3\)
30	\(π/6\)	135	\(3π/4\)
45	\(π/4\)	150	\(5π/6\)
60	\(π/3\)	180	\(π\)
90	\(π/2\)