Skip to main content
Global

3.5：间隔表示法和无穷大

  1. Last updated
  2. Save as PDF
  • Page ID
    171032

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    什么是无限？ 无穷不是一个实数。 Infinity 比任何可以想象的数字都要大。 这是一个没有界限的想法。 直线是无界限的示例。 例如，数字线的末端有箭头来表示这种无界限的想法。 用于表示无穷大的符号是\(\infty\)。 数字行的左侧是\(−\infty\)，数字行的右侧\(\infty\)用于描述数字线的无限行为。

    间隔符号 数字线
    a)\((12,\infty)\)
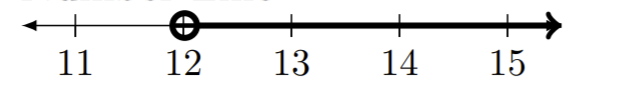
    clipboard_e80433e1e62fd9286767d7fccd338cf9b.png
    图 Template:Index
    b)\([-5,\infty)\)
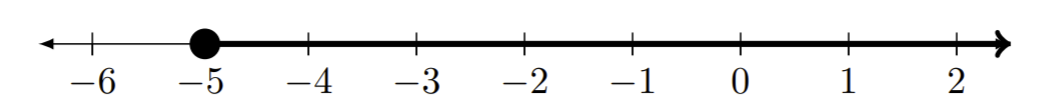
    clipboard_eb315c734eff17646d4b6150afe365542.png
    图 Template:Index
    c)\((−\infty,4)\)
    clipboard_e347ccb8b609ce4a6a16613afe5ef2c4b.png
    图 Template:Index
    d)\((−\infty,0]\)
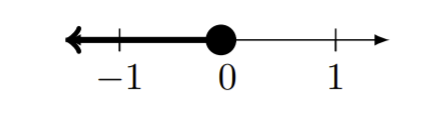
    clipboard_e960bf6751735e85b398571075c5f0bf9.png
    图 Template:Index
    e)\((−\infty,\infty)\)
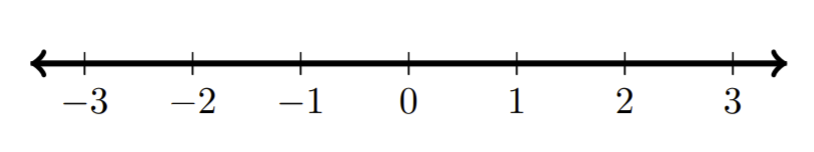
    clipboard_e65f0217f121cc70ff0562beb8ad1573f.png
    图 Template:Index

    注意：由于\(\infty\)不是实数，因此必须使用圆括号 (,)。 \(\infty\)不能包含在间隔中。

    练习 Template:Index

    画一条与以下间隔相对应的数字线。

    1. \((−\infty,5)\)
    2. \([-5,\infty)\)
    3. \((−\infty,\infty)\)
    4. \((-3,\infty)\)
    5. \((−\infty,-2]\)