10.3: Transições de fase
- Page ID
- 184780
- Defina transições de fase e temperaturas de transição de fase
- Explicar a relação entre temperaturas de transição de fase e forças de atração intermoleculares
- Descreva os processos representados pelas curvas típicas de aquecimento e resfriamento e calcule os fluxos de calor e as mudanças de entalpia que acompanham esses processos
Testemunhamos e utilizamos mudanças de estado físico, ou transições de fase, de várias maneiras. Como um exemplo de importância global, considere a evaporação, condensação, congelamento e derretimento da água. Essas mudanças de estado são aspectos essenciais do ciclo da água na Terra, bem como muitos outros fenômenos naturais e processos tecnológicos de importância central para nossas vidas. Neste módulo, os aspectos essenciais das transições de fase são explorados.
Vaporização e condensação
Quando um líquido se vaporiza em um recipiente fechado, as moléculas de gás não conseguem escapar. À medida que essas moléculas da fase gasosa se movem aleatoriamente, elas ocasionalmente colidem com a superfície da fase condensada e, em alguns casos, essas colisões resultarão na reentrada das moléculas na fase condensada. A mudança da fase gasosa para o líquido é chamada de condensação. Quando a taxa de condensação se torna igual à taxa de vaporização, nem a quantidade de líquido nem a quantidade de vapor no recipiente mudam. Diz-se então que o vapor no recipiente está em equilíbrio com o líquido. Lembre-se de que essa não é uma situação estática, pois as moléculas são trocadas continuamente entre as fases condensada e gasosa. Esse é um exemplo de equilíbrio dinâmico, o status de um sistema no qual processos recíprocos (por exemplo, vaporização e condensação) ocorrem em taxas iguais. A pressão exercida pelo vapor em equilíbrio com um líquido em um recipiente fechado a uma determinada temperatura é chamada de pressão de vapor do líquido (ou pressão de vapor de equilíbrio). A área da superfície do líquido em contato com o vapor e o tamanho do vaso não afetam a pressão do vapor, embora afetem o tempo necessário para que o equilíbrio seja alcançado. Podemos medir a pressão de vapor de um líquido colocando uma amostra em um recipiente fechado, como o ilustrado na Figura\(\PageIndex{1}\), e usando um manômetro para medir o aumento da pressão devido ao vapor em equilíbrio com a fase condensada.
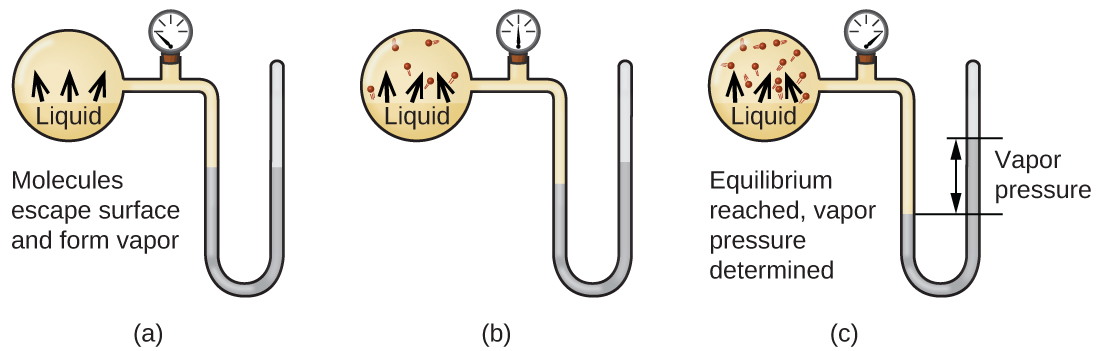
As identidades químicas das moléculas em um líquido determinam os tipos (e forças) de atrações intermoleculares possíveis; consequentemente, diferentes substâncias exibirão diferentes pressões de vapor de equilíbrio. Forças atrativas intermoleculares relativamente fortes servirão para impedir a vaporização, bem como favorecer a “recaptura” de moléculas em fase gasosa quando elas colidem com a superfície do líquido, resultando em uma pressão de vapor relativamente baixa. As atrações intermoleculares fracas apresentam menos barreira à vaporização e uma probabilidade reduzida de recaptura de gás, produzindo pressões de vapor relativamente altas. O exemplo a seguir ilustra essa dependência da pressão de vapor nas forças de atração intermoleculares.
Dadas as fórmulas estruturais mostradas para esses quatro compostos, explique suas pressões relativas de vapor em termos de tipos e extensões de IMFs:
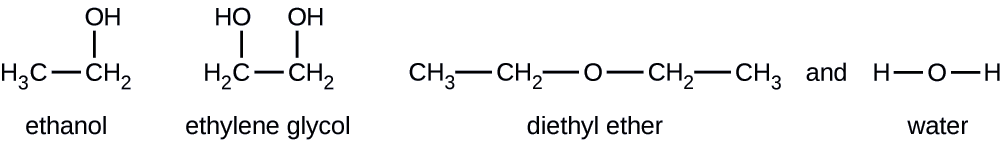
Solução
O éter dietílico tem um dipolo muito pequeno e a maioria de suas atrações intermoleculares são as forças de Londres. Embora essa molécula seja a maior das quatro consideradas, seus FMI são os mais fracos e, como resultado, suas moléculas escapam mais facilmente do líquido. Ele também tem a maior pressão de vapor. Devido ao seu tamanho menor, o etanol apresenta forças de dispersão mais fracas do que o éter dietílico. No entanto, o etanol é capaz de se ligar ao hidrogênio e, portanto, exibe FMI gerais mais fortes, o que significa que menos moléculas escapam do líquido a qualquer temperatura e, portanto, o etanol tem uma pressão de vapor menor do que o éter dietílico. A água é muito menor do que qualquer uma das substâncias anteriores e exibe forças de dispersão mais fracas, mas sua extensa ligação de hidrogênio fornece atrações intermoleculares mais fortes, menos moléculas escapando do líquido e uma pressão de vapor menor do que a do éter dietílico ou do etanol. O etilenoglicol tem dois grupos −OH, então, como a água, ele exibe uma extensa ligação de hidrogênio. É muito maior do que a água e, portanto, experimenta forças maiores de Londres. Seus FMI gerais são as maiores dessas quatro substâncias, o que significa que sua taxa de vaporização será a mais lenta e, consequentemente, sua pressão de vapor a mais baixa.
At 20 °C, the vapor pressures of several alcohols are given in this table. Explain these vapor pressures in terms of types and extents of IMFs for these alcohols:
| Compound | methanol CH3OH | ethanol C2H5OH | propanol C3H7OH | butanol C4H9OH |
|---|---|---|---|---|
| Vapor Pressure at 20 °C | 11.9 kPa | 5.95 kPa | 2.67 kPa | 0.56 kPa |
- Answer
-
All these compounds exhibit hydrogen bonding; these strong IMFs are difficult for the molecules to overcome, so the vapor pressures are relatively low. As the size of molecule increases from methanol to butanol, dispersion forces increase, which means that the vapor pressures decrease as observed:
\[P_{methanol} > P_{ethanol} > P_{propanol} > P_{butanol} \nonumber \]
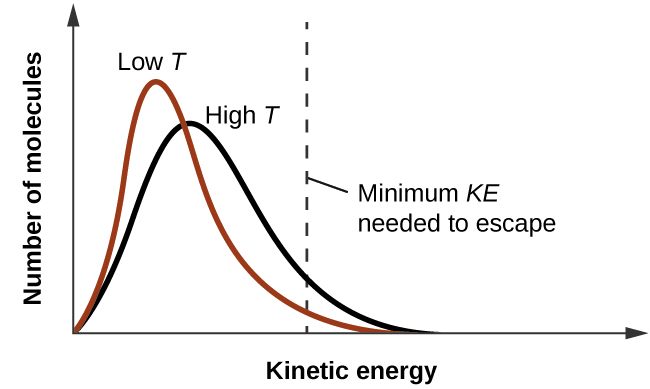
As temperature increases, the vapor pressure of a liquid also increases due to the increased average KE of its molecules. Recall that at any given temperature, the molecules of a substance experience a range of kinetic energies, with a certain fraction of molecules having a sufficient energy to overcome IMF and escape the liquid (vaporize). At a higher temperature, a greater fraction of molecules have enough energy to escape from the liquid, as shown in Figure \(\PageIndex{2}\). The escape of more molecules per unit of time and the greater average speed of the molecules that escape both contribute to the higher vapor pressure.
Boiling Points
When the vapor pressure increases enough to equal the external atmospheric pressure, the liquid reaches its boiling point. The boiling point of a liquid is the temperature at which its equilibrium vapor pressure is equal to the pressure exerted on the liquid by its gaseous surroundings. For liquids in open containers, this pressure is that due to the earth’s atmosphere. The normal boiling point of a liquid is defined as its boiling point when surrounding pressure is equal to 1 atm (101.3 kPa). Figure \(\PageIndex{3}\) shows the variation in vapor pressure with temperature for several different substances. Considering the definition of boiling point, these curves may be seen as depicting the dependence of a liquid’s boiling point on surrounding pressure.

A typical atmospheric pressure in Leadville, Colorado (elevation 10,200 feet) is 68 kPa. Use the graph in Figure \(\PageIndex{3}\) to determine the boiling point of water at this elevation.
Solution
The graph of the vapor pressure of water versus temperature in Figure \(\PageIndex{3}\) indicates that the vapor pressure of water is 68 kPa at about 90 °C. Thus, at about 90 °C, the vapor pressure of water will equal the atmospheric pressure in Leadville, and water will boil.
The boiling point of ethyl ether was measured to be 10 °C at a base camp on the slopes of Mount Everest. Use Figure \(\PageIndex{3}\) to determine the approximate atmospheric pressure at the camp.
- Answer
-
Approximately 40 kPa (0.4 atm)
The quantitative relation between a substance’s vapor pressure and its temperature is described by the Clausius-Clapeyron equation:
\[P=Ae^{−ΔH_{vap}/RT} \label{10.4.1} \]
where
- \(ΔH_{vap}\) is the enthalpy of vaporization for the liquid,
- \(R\) is the gas constant, and
- \(\ln A\) is a constant whose value depends on the chemical identity of the substance.
Equation \(\ref{10.4.1}\) is often rearranged into logarithmic form to yield the linear equation:
\[\ln P=−\dfrac{ΔH_\ce{vap}}{RT}+\ln A \label{10.4.2} \]
This linear equation may be expressed in a two-point format that is convenient for use in various computations, as demonstrated in the examples and exercises that follow. If at temperature \(T_1\), the vapor pressure is \( P_1\), and at temperature \(T_2\), the vapor pressure is \(T_2\), the corresponding linear equations are:
\[\ln P_1=−\dfrac{ΔH_\ce{vap}}{RT_1}+\ln A \nonumber \]
and
\[\ln P_2=−\dfrac{ΔH_\ce{vap}}{RT_2}+\ln A \label{10.4.3} \]
Since the constant, ln A, is the same, these two equations may be rearranged to isolate \(\ln A\) and then set them equal to one another:
\(\ln P_1+\dfrac{ΔH_\ce{vap}}{RT_1}=\ln P_2+\dfrac{ΔH_\ce{vap}}{RT_2}\label{10.4.5}\)
which can be combined into:
\[\ln \left(\dfrac{P_2}{P_1}\right)=\dfrac{ΔH_\ce{vap}}{R} \left( \dfrac{1}{T_1}−\dfrac{1}{T_2}\right) \label{10.4.6} \]
Isooctane (2,2,4-trimethylpentane) has an octane rating of 100. It is used as one of the standards for the octane-rating system for gasoline. At 34.0 °C, the vapor pressure of isooctane is 10.0 kPa, and at 98.8 °C, its vapor pressure is 100.0 kPa. Use this information to estimate the enthalpy of vaporization for isooctane.
Solution
The enthalpy of vaporization, \(ΔH_\ce{vap}\), can be determined by using the Clausius-Clapeyron equation (Equation \(\ref{10.4.6}\)):
\[\ln\left(\dfrac{P_2}{P_1}\right)=\dfrac{ΔH_\ce{vap}}{R}\left(\dfrac{1}{T_1}−\dfrac{1}{T_2}\right) \nonumber \]
Since we have two vapor pressure-temperature values
- \(T_1 = 34.0^oC = 307.2\, K\)
- \(P_1 = 10.0\, kPa\) and
- \(T_2 = 98.8 ^oC = 372.0\, K\)
- \(P_2 = 100\, kPa\)
we can substitute them into this equation and solve for \(ΔH_{vap}\). Rearranging the Clausius-Clapeyron equation and solving for \(ΔH_{vap}\) yields:
\[ \begin{align*} ΔH_\ce{vap} &= \dfrac{R⋅\ln\left(\dfrac{P_2}{P_1}\right)}{\left(\dfrac{1}{T_1}−\dfrac{1}{T_2}\right)} \\[4pt] &= \dfrac{(8.3145\:J/mol⋅K)⋅\ln \left(\dfrac{100\: kPa}{10.0\: kPa}\right)}{\left(\dfrac{1}{307.2\:K}−\dfrac{1}{372.0\:K}\right)} \\[4pt] &=33,800\, J/mol =33.8\, kJ/mol \end{align*} \nonumber \]
Note that the pressure can be in any units, so long as they agree for both P values, but the temperature must be in kelvin for the Clausius-Clapeyron equation to be valid.
At 20.0 °C, the vapor pressure of ethanol is 5.95 kPa, and at 63.5 °C, its vapor pressure is 53.3 kPa. Use this information to estimate the enthalpy of vaporization for ethanol.
- Answer
-
47,782 J/mol = 47.8 kJ/mol
For benzene (C6H6), the normal boiling point is 80.1 °C and the enthalpy of vaporization is 30.8 kJ/mol. What is the boiling point of benzene in Denver, where atmospheric pressure = 83.4 kPa?
Solution
If the temperature and vapor pressure are known at one point, along with the enthalpy of vaporization, ΔHvap, then the temperature that corresponds to a different vapor pressure (or the vapor pressure that corresponds to a different temperature) can be determined by using the Clausius-Clapeyron equation (Equation \(\ref{10.4.1}\)) :
\[\ln\left(\dfrac{P_2}{P_1}\right)=\dfrac{ΔH_\ce{vap}}{R}\left(\dfrac{1}{T_1}−\dfrac{1}{T_2}\right) \nonumber \]
Since the normal boiling point is the temperature at which the vapor pressure equals atmospheric pressure at sea level, we know one vapor pressure-temperature value (\(T_1\) = 80.1 °C = 353.3 K, \(P_1\) = 101.3 kPa, \(ΔH_{vap}\) = 30.8 kJ/mol) and want to find the temperature (\(T_2\)) that corresponds to vapor pressure P2 = 83.4 kPa. We can substitute these values into the Clausius-Clapeyron equation and then solve for \(T_2\). Rearranging the Clausius-Clapeyron equation and solving for \(T_2\) yields:
\[\begin{align*}
T_2 &=\left(\dfrac{−R⋅\ln\left(\dfrac{P_2}{P_1}\right)}{ΔH_\ce{vap}}+\dfrac{1}{T_1}\right)^{−1} \\[4pt] &=\mathrm{\left(\dfrac{−(8.3145\:J/mol⋅K)⋅\ln\left(\dfrac{83.4\:kPa}{101.3\:kPa}\right)}{30,800\: J/mol}+\dfrac{1}{353.3\:K}\right)^{−1}}\\[4pt]
&=\mathrm{346.9\: K\: or\:73.8^\circ C}
\end{align*} \nonumber \]
For acetone \(\ce{(CH3)2CO}\), the normal boiling point is 56.5 °C and the enthalpy of vaporization is 31.3 kJ/mol. What is the vapor pressure of acetone at 25.0 °C?
- Answer
-
30.1 kPa
Enthalpy of Vaporization
Vaporization is an endothermic process. The cooling effect can be evident when you leave a swimming pool or a shower. When the water on your skin evaporates, it removes heat from your skin and causes you to feel cold. The energy change associated with the vaporization process is the enthalpy of vaporization, \(ΔH_{vap}\). For example, the vaporization of water at standard temperature is represented by:
\[\ce{H2O}(l)⟶\ce{H2O}(g)\hspace{20px}ΔH_\ce{vap}=\mathrm{44.01\: kJ/mol} \nonumber \]
As described in the chapter on thermochemistry, the reverse of an endothermic process is exothermic. And so, the condensation of a gas releases heat:
\[\ce{H2O}(g)⟶\ce{H2O}(l)\hspace{20px}ΔH_\ce{con}=−ΔH_\ce{vap}=\mathrm{−44.01\:kJ/mol} \nonumber \]
One way our body is cooled is by evaporation of the water in sweat (Figure \(\PageIndex{4}\)). In very hot climates, we can lose as much as 1.5 L of sweat per day. Although sweat is not pure water, we can get an approximate value of the amount of heat removed by evaporation by assuming that it is. How much heat is required to evaporate 1.5 L of water (1.5 kg) at T = 37 °C (normal body temperature); \(ΔH_{vap} = 43.46\, kJ/mol\) at 37 °C.

Solution We start with the known volume of sweat (approximated as just water) and use the given information to convert to the amount of heat needed:
\[\mathrm{1.5\cancel{L}×\dfrac{1000\cancel{g}}{1\cancel{L}}×\dfrac{1\cancel{mol}}{18\cancel{g}}×\dfrac{43.46\:kJ}{1\cancel{mol}}=3.6×10^3\:kJ} \nonumber \]
Thus, 3600 kJ of heat are removed by the evaporation of 1.5 L of water.
How much heat is required to evaporate 100.0 g of liquid ammonia, \(\ce{NH3}\), at its boiling point if its enthalpy of vaporization is 4.8 kJ/mol?
- Answer
-
28 kJ
Melting and Freezing
When we heat a crystalline solid, we increase the average energy of its atoms, molecules, or ions and the solid gets hotter. At some point, the added energy becomes large enough to partially overcome the forces holding the molecules or ions of the solid in their fixed positions, and the solid begins the process of transitioning to the liquid state, or melting. At this point, the temperature of the solid stops rising, despite the continual input of heat, and it remains constant until all of the solid is melted. Only after all of the solid has melted will continued heating increase the temperature of the liquid (Figure \(\PageIndex{5}\).
If we stop heating during melting and place the mixture of solid and liquid in a perfectly insulated container so no heat can enter or escape, the solid and liquid phases remain in equilibrium. This is almost the situation with a mixture of ice and water in a very good thermos bottle; almost no heat gets in or out, and the mixture of solid ice and liquid water remains for hours. In a mixture of solid and liquid at equilibrium, the reciprocal processes of melting and freezing occur at equal rates, and the quantities of solid and liquid therefore remain constant. The temperature at which the solid and liquid phases of a given substance are in equilibrium is called the melting point of the solid or the freezing point of the liquid. Use of one term or the other is normally dictated by the direction of the phase transition being considered, for example, solid to liquid (melting) or liquid to solid (freezing).
The enthalpy of fusion and the melting point of a crystalline solid depend on the strength of the attractive forces between the units present in the crystal. Molecules with weak attractive forces form crystals with low melting points. Crystals consisting of particles with stronger attractive forces melt at higher temperatures.
The amount of heat required to change one mole of a substance from the solid state to the liquid state is the enthalpy of fusion, ΔHfus of the substance. The enthalpy of fusion of ice is 6.0 kJ/mol at 0 °C. Fusion (melting) is an endothermic process:
\[\ce{H2O}_{(s)} \rightarrow \ce{H2O}_{(l)} \;\; ΔH_\ce{fus}=\mathrm{6.01\; kJ/mol} \label{10.4.9} \]
The reciprocal process, freezing, is an exothermic process whose enthalpy change is −6.0 kJ/mol at 0 °C:
\[\ce{H_2O}_{(l)} \rightarrow \ce{H_2O}_{(s)}\;\; ΔH_\ce{frz}=−ΔH_\ce{fus}=−6.01\;\mathrm{kJ/mol} \label{10.4.10} \]
Sublimation and Deposition
Some solids can transition directly into the gaseous state, bypassing the liquid state, via a process known as sublimation. At room temperature and standard pressure, a piece of dry ice (solid CO2) sublimes, appearing to gradually disappear without ever forming any liquid. Snow and ice sublime at temperatures below the melting point of water, a slow process that may be accelerated by winds and the reduced atmospheric pressures at high altitudes. When solid iodine is warmed, the solid sublimes and a vivid purple vapor forms (Figure \(\PageIndex{6}\)). The reverse of sublimation is called deposition, a process in which gaseous substances condense directly into the solid state, bypassing the liquid state. The formation of frost is an example of deposition.

Like vaporization, the process of sublimation requires an input of energy to overcome intermolecular attractions. The enthalpy of sublimation, ΔHsub, is the energy required to convert one mole of a substance from the solid to the gaseous state. For example, the sublimation of carbon dioxide is represented by:
\[\ce{CO2}(s)⟶\ce{CO2}(g)\hspace{20px}ΔH_\ce{sub}=\mathrm{26.1\: kJ/mol} \nonumber \]
Likewise, the enthalpy change for the reverse process of deposition is equal in magnitude but opposite in sign to that for sublimation:
\[\ce{CO2}(g)⟶\ce{CO2}(s)\hspace{20px}ΔH_\ce{dep}=−ΔH_\ce{sub}=\mathrm{−26.1\:kJ/mol} \nonumber \]
Consider the extent to which intermolecular attractions must be overcome to achieve a given phase transition. Converting a solid into a liquid requires that these attractions be only partially overcome; transition to the gaseous state requires that they be completely overcome. As a result, the enthalpy of fusion for a substance is less than its enthalpy of vaporization. This same logic can be used to derive an approximate relation between the enthalpies of all phase changes for a given substance. Though not an entirely accurate description, sublimation may be conveniently modeled as a sequential two-step process of melting followed by vaporization in order to apply Hess’s Law.
\[\mathrm{solid⟶liquid}\hspace{20px}ΔH_\ce{fus}\\\underline{\mathrm{liquid⟶gas}\hspace{20px}ΔH_\ce{vap}}\\\mathrm{solid⟶gas}\hspace{20px}ΔH_\ce{sub}=ΔH_\ce{fus}+ΔH_\ce{vap} \nonumber \]
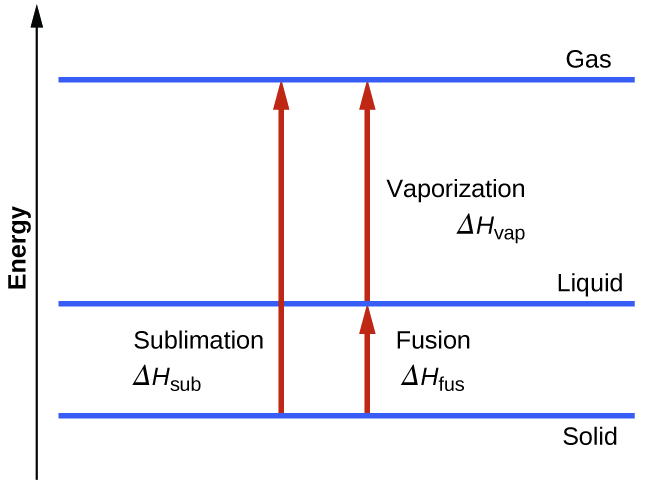
Heating and Cooling Curves
In the chapter on thermochemistry, the relation between the amount of heat absorbed or related by a substance, q, and its accompanying temperature change, ΔT, was introduced:
\[q=mcΔT \nonumber \]
where m is the mass of the substance and c is its specific heat. The relation applies to matter being heated or cooled, but not undergoing a change in state. When a substance being heated or cooled reaches a temperature corresponding to one of its phase transitions, further gain or loss of heat is a result of diminishing or enhancing intermolecular attractions, instead of increasing or decreasing molecular kinetic energies. While a substance is undergoing a change in state, its temperature remains constant. Figure \(\PageIndex{8}\) shows a typical heating curve.
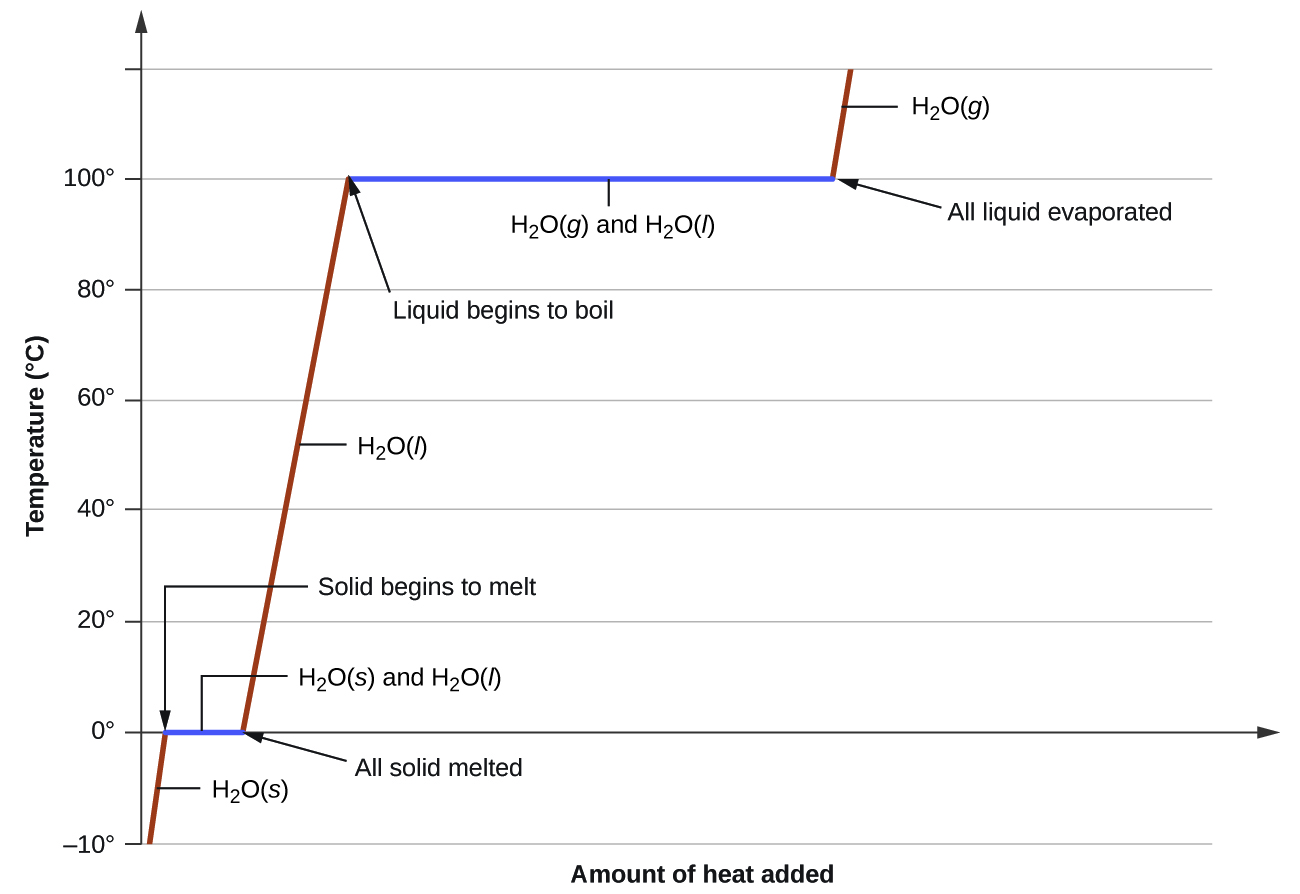
Consider the example of heating a pot of water to boiling. A stove burner will supply heat at a roughly constant rate; initially, this heat serves to increase the water’s temperature. When the water reaches its boiling point, the temperature remains constant despite the continued input of heat from the stove burner. This same temperature is maintained by the water as long as it is boiling. If the burner setting is increased to provide heat at a greater rate, the water temperature does not rise, but instead the boiling becomes more vigorous (rapid). This behavior is observed for other phase transitions as well: For example, temperature remains constant while the change of state is in progress.
How much heat is required to convert 135 g of ice at −15 °C into water vapor at 120 °C?
Solution
The transition described involves the following steps:
- Heat ice from −15 °C to 0 °C
- Melt ice
- Heat water from 0 °C to 100 °C
- Boil water
- Heat steam from 100 °C to 120 °C
The heat needed to change the temperature of a given substance (with no change in phase) is: q = m × c × ΔT (see previous chapter on thermochemistry). The heat needed to induce a given change in phase is given by q = n × ΔH.
Using these equations with the appropriate values for specific heat of ice, water, and steam, and enthalpies of fusion and vaporization, we have:
\[\begin{align*}
q_\ce{total}&=(m⋅c⋅ΔT)_\ce{ice}+n⋅ΔH_\ce{fus}+(m⋅c⋅ΔT)_\ce{water}+n⋅ΔH_\ce{vap}+(m⋅c⋅ΔT)_\ce{steam}\\[7pt]
&=\mathrm{(135\: g⋅2.09\: J/g⋅°C⋅15°C)+\left(135⋅\dfrac{1\: mol}{18.02\:g}⋅6.01\: kJ/mol
\right)}\\[7pt]
&\mathrm{+(135\: g⋅4.18\: J/g⋅°C⋅100°C)+\left(135\: g⋅\dfrac{1\: mol}{18.02\:g}⋅40.67\: kJ/mol\right)}\\[7pt]
&\mathrm{+(135\: g⋅1.84\: J/g⋅°C⋅20°C)}\\[7pt]
&=\mathrm{4230\: J+45.0\: kJ+56,500\: J+305\: kJ+4970\: J}
\end{align*} \nonumber \]
Converting the quantities in J to kJ permits them to be summed, yielding the total heat required:
\[\mathrm{=4.23\:kJ+45.0\: kJ+56.5\: kJ+305\: kJ+4.97\: kJ=416\: kJ} \nonumber \]
What is the total amount of heat released when 94.0 g water at 80.0 °C cools to form ice at −30.0 °C?
- Answer
-
40.5 kJ
Summary
Phase transitions are processes that convert matter from one physical state into another. There are six phase transitions between the three phases of matter. Melting, vaporization, and sublimation are all endothermic processes, requiring an input of heat to overcome intermolecular attractions. The reciprocal transitions of freezing, condensation, and deposition are all exothermic processes, involving heat as intermolecular attractive forces are established or strengthened. The temperatures at which phase transitions occur are determined by the relative strengths of intermolecular attractions and are, therefore, dependent on the chemical identity of the substance.
Key Equations
- \(P=Ae^{−ΔH_\ce{vap}/RT}\)
- \(\ln P=−\dfrac{ΔH_\ce{vap}}{RT}+\ln A\)
- \(\ln\left(\dfrac{P_2}{P_1}\right)=\dfrac{ΔH_\ce{vap}}{R}\left(\dfrac{1}{T_1}−\dfrac{1}{T_2}\right)\)
Glossary
- boiling point
- temperature at which the vapor pressure of a liquid equals the pressure of the gas above it
- Clausius-Clapeyron equation
- mathematical relationship between the temperature, vapor pressure, and enthalpy of vaporization for a substance
- condensation
- change from a gaseous to a liquid state
- deposition
- change from a gaseous state directly to a solid state
- dynamic equilibrium
- state of a system in which reciprocal processes are occurring at equal rates
- freezing
- change from a liquid state to a solid state
- freezing point
- temperature at which the solid and liquid phases of a substance are in equilibrium; see also melting point
- melting
- change from a solid state to a liquid state
- melting point
- temperature at which the solid and liquid phases of a substance are in equilibrium; see also freezing point
- normal boiling point
- temperature at which a liquid’s vapor pressure equals 1 atm (760 torr)
- sublimation
- change from solid state directly to gaseous state
- vapor pressure
- (also, equilibrium vapor pressure) pressure exerted by a vapor in equilibrium with a solid or a liquid at a given temperature
- vaporization
- change from liquid state to gaseous state


