10.3: 理性不平等

求解有理不等式需要找到分子和分母的零，然后使用这些值调查数字线上的解集区域。

示例 Template:Index

求解不等式并用区间表示法写出解集：

1. $\dfrac{x − 1}{x + 1} ≥ 0$
2. $\dfrac{2x − 3}{x + 1} ≤ 0$
3. $\dfrac{x + 2}{x − 2} ≥ 0$

解决方案

1. $\begin{array} &&\dfrac{x − 1}{x + 1} ≥ 0 &\text{Example problem} \\ &\dfrac{x − 1}{x + 1} ≥ 0 &\text{The quotient must be greater than or equal to \(0$.}\\ &x − 1 = 0,\; x = 1 &\ text {找到分子的零}\\ &x + 1 = 0, x =\; −1 &\ text {找到分母的零}\ end {array}\)

零将数字行划分为多个$3$区域，$x < −1$，$−1 < x < 1$，$x > 1$

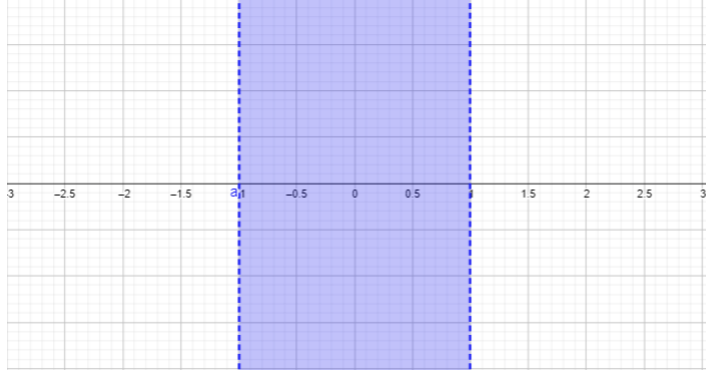
$\begin{array} &&\text{For } x < −1, \text{ choose } x = −2. \;\;\dfrac{−2 − 1}{−2 + 1} = \dfrac{−3}{−1} = 3 ≥ 0 \\ &\text{Replacing \(-2$表示答案中的$x$结果$3$，该结果大于或等于$0$。 $x < −1$该区域包含在解决方案集中。}\\ [0.25in] &\ text {For} −1 < x < 1，\ text {choose} x = 0。\;\ dfrac {0 − 1} {0 + 1} = −1 < 0\\ &\ text {替换$0$答案中的$x$结果$-1$，小于$0$，不满足问题中给定的不等式。}\\ &\ text {此区域$−1 < x < 1$不包括在解集中。}\\ [0.25in] &\ text {For} x > 1，\ text {choose} x = 2.\;\;\ dfrac {2 + 1} {2 + 1} =\ dfrac {1} ≥ 0\\ &\ text {替换$2$答案中的$x$结果$\dfrac{1}{3}$，哪个是大于或等于$0$。 $x > 1$该区域包含在解集中。}\\ [0.25in] & (−∞, −1) (1, ∞)\\ &\ text {用区间表示法书写的最终答案（有关更多详细信息，请参阅区间表示法部分）。} \ end {array}\)

2. $\begin{array} &&\dfrac{2x − 3}{x + 1} ≤ 0 &\text{Example problem} \\ &\dfrac{2x − 3}{x + 1} ≤ 0 &\text{The quotient must be less than or equal to \(0$.}\\ &2x − 3 = 0,\; x = 1.5 &\ text {找到分子的零}\\ &x + 1 = 0,\; x = −1 &\ text {找到分母的零}\ end {array}\)

零将数字行划分为多个$3$区域，$x < −1$，$−1 < x < 1.5$，$x > 1.5$

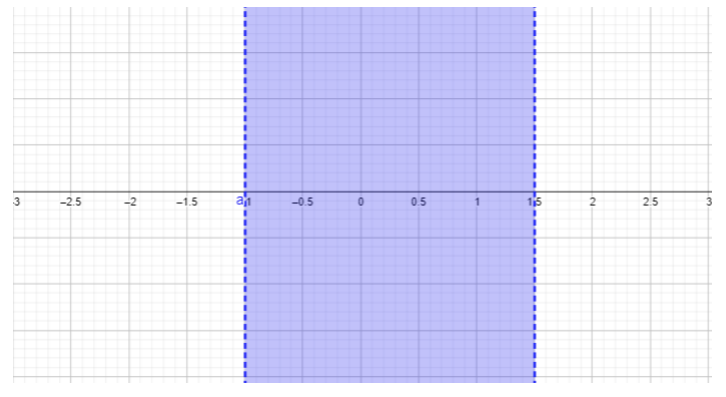
$\begin{array} &&\text{For } x < −1, \text{ choose } x = −2. \;\; \dfrac{2(−2) − 3}{−2 − 1} = \dfrac{−7}{−3} = \dfrac{7}{3} ≥ 0 \\ &\text{Replacing \(-2$表示答案中的$x$结果大于$\dfrac{7}{3}$$0$，未满足问题中给定的不等式。}\\ &\ text {此区域$x < −1$不在解集合中。}\\ [0.25in] &\ text {For} −1 < x < 1.5，\ text {choose} x = 0。\;\ dfrac {2 (0) −3} {0 − 1} =\ dfrac {−3} {−1} = 3 ≥ 0\\ &\ text {替换$0$答案中的$x$结果$3$，大于或等于$0$，这不是问题所要求的。}\\ &\ text {解集中不包括该区域$−1 < x < 1.5$。}\\ [0.25in] &\ text {For} x > 1，\ text {choose} x = 2。\;\ dfrac {2 (2) − 3} {2 − 1} =\ dfrac {1} {1} = 1 ≥ 0\\ &\ text {替换$2$答案中的$x$结果$1$，大于或等于$0$。 该区域$x > 1$不包含在解决方案集中。}\\ [0.25in] &\\ &\ text {这个问题没有解决方案。 $\dfrac{2x − 3}{x + 1}$永远不会小于或等于$0$。} \ end {array}\)

3. $\begin{array} &&\dfrac{x + 2}{x − 2} ≥ 0 &\text{Example problem} \\ &\dfrac{x + 2}{x − 2} ≥ 0 &\text{The quotient must be greater than or equal to \(0$.}\\ &x + 2 = 0,\;\; x = −2 &\ text {找到分子的零}\\ &x − 2 = 0,\;\; x = 2 &\ text {找到分母的零}\ end {array}\)

零将数字行划分为多个$3$区域，$x < −2$，$−2 < x < 2$，$x > 2$

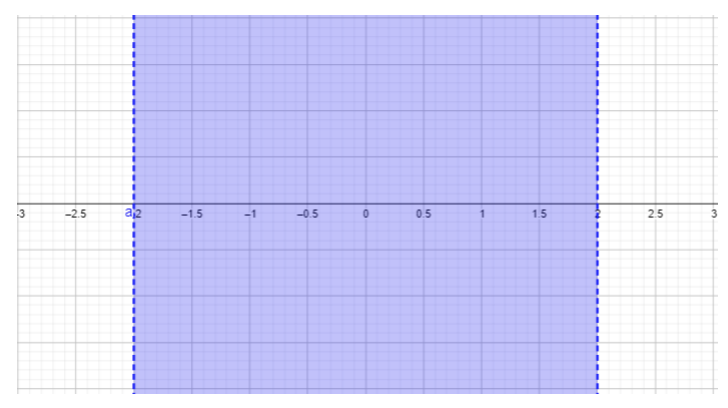
$\begin{array} &&\text{For } x < −2, \text{ choose } x = −3. \dfrac{−3 + 2}{−3 − 2} = \dfrac{−1}{−5} = \dfrac{1}{5} ≥ 0 \\ &\text{Replacing \(-3$表示答案中的$x$结果$\dfrac{1}{5}$，该结果大于或等于$0$。 $x < −2$该区域包含在解决方案集中。}\\ [0.25in] &\ text {For} −2 < x < 2，\ text {choose} x = 0。\;\ dfrac {0 + 2} {0 − 2} = −1 < 0\\ &\ text {替换$0$答案中的$x$结果$-1$，小于$0$，不满足问题中给定的不等式。}\\ &\ text {此区域$−2 < x < 2$不包含在解集中。}\\ [0.25in] &\ text {For} x > 2，\ text {choose} x = 3.\;\ dfrac {3 + 2} {3 − 2} =\ dfrac {5} {1} = 5 ≥ 0\\ &\ text {替换$3$$x$结果为回答$5$，它大于或等于$0$。 $x > 2$该区域包含在解集中。}\\ [0.25in] & (−∞, −2) (2, ∞)\\ &\ text {用区间表示法书写的最终答案（更多详情请参阅区间表示法部分）。} \ end {array}\)

练习 Template:Index

1. $\dfrac{x + 3}{x − 2} ≥ 0$
2. $\dfrac{x − 2}{x − 1} ≤ 0$
3. $\dfrac{2x − 1}{x + 2} ≤ 0$
4. $\dfrac{2x − 3}{x + 1} ≥ 0$